a3+b3 Formula: Check a3-b3 Formula, a^3-b^3, a ^ 3 + b ^ 3 Formula Solutions

The a3-b3 formula is used for the calculation of the difference between two cubes and a3−b3 formula is = (a−b)(a2 + b2 + ab). On the other hand, the a3+b3 formula is a3 + b3 = 3ab(a + b) – (a + b)3 or a3 + b3 = (a2 – ab + b2)(a + b). It is one of the most important formulas in algebra and this formula finds its application in many fields. In this article, we will explore different aspects of this formula including its proof.

a3 b3 Formula Algebraic Equation

An algebraic equation, often known as a polynomial equation, is a mathematical equation of the form:

P = 0

P is a polynomial having coefficients in a field, most commonly the field of rational numbers. Many authors solely use the word algebraic equation to describe univariate equations, or polynomial equations with only one variable. A polynomial equation, on the other hand, can have several variables. Polynomial equation is frequently favored over algebraic equation when there are multiple variables (multivariate scenario). These equations consist of constant terms (1, 2, 3) and variable terms (x, y, z) raised to some powers or a combination of both. Some of the examples of algebraic equations are 4x + 3, 7 – 2x², 4x² + 3x.

a³ b³ Formulas

Some a3 b3 Formulas are given below.

• (a + b)³ = a³ + b³ + 3ab (a + b)
• (a – b)³ = a³ – b³ – 3ab (a-b)
• a³ + b³ = (a + b) (a² – ab + b²)
• a³ – b³ = (a – b) (a² + ab + b²)
• a³ + b³ + c³ – 3abc = (a + b + c) (a² + b² + c² – ab – bc – ca)
• a + b = (a³ + b³ ) / (a² – ab + b²)

a3-b3 Formula – a^3-b^3 Formula

The formula you are referring to, “a3 – b3,” represents the difference of cubes. It is an algebraic identity that can be factored into a product of binomials. The formula is as follows:

a^3 – b^3 = (a – b)(a^2 + ab + b^2)

This formula can be derived by expanding the expression (a – b)(a^2 + ab + b^2) using the distributive property. The result of the expansion will be a^3 – ab^2 + a^2b – b^3. Notice that the middle terms, -ab^2 and a^2b, cancel each other out, leaving only a^3 – b^3.

The a3-b3 formula for the difference of cubes is useful in algebraic simplification and factoring. It allows you to factor expressions that are in the form of a difference of cubes, making it easier to work with and solve equations involving such expressions.

a3+b3 Formula – a^3+b^3 Formula

The formula you are referring to, “a3 + b3,” represents the sum of cubes. Similar to the difference of cubes formula, it can be factored into a product of binomials. The formula is as follows:

a^3 + b^3 = (a + b)(a^2 – ab + b^2)

This formula can be derived by expanding the expression (a + b)(a^2 – ab + b^2) using the distributive property. The result of the expansion will be a^3 + ab^2 + a^2b + b^3. Notice that the middle terms, ab^2 and a^2b, cancel each other out, leaving only a^3 + b^3.

The formula for the sum of cubes is useful in algebraic simplification and factoring. It allows you to factor expressions that are in the form of a sum of cubes, making it easier to work with and solve equations involving such expressions.

(a+b)^3 Formula

The formula you are referring to, “(a + b)^3,” represents the cube of a binomial. It can be expanded using the binomial theorem. The formula is as follows:

(a + b)^3 = a^3 + 3a^2b + 3ab^2 + b^3

This formula can be derived using the binomial theorem, which states that for any positive integer n:

(a + b)^n = C(n, 0)a^n + C(n, 1)a^(n-1)b + C(n, 2)a^(n-2)b^2 + … + C(n, n-1)ab^(n-1) + C(n, n)b^n

In the case of (a + b)^3, where n = 3, the binomial coefficients are as follows:

C(3, 0) = 1 C(3, 1) = 3 C(3, 2) = 3 C(3, 3) = 1

By substituting these coefficients into the formula, we get:

(a + b)^3 = 1a^3 + 3a^2b + 3ab^2 + 1b^3

Simplifying, we obtain the expanded form of (a + b)^3:

(a + b)^3 = a^3 + 3a^2b + 3ab^2 + b^3

The formula for the cube of a binomial, or the expanded form of (a + b)^3, allows you to expand and simplify expressions involving cubed binomials.

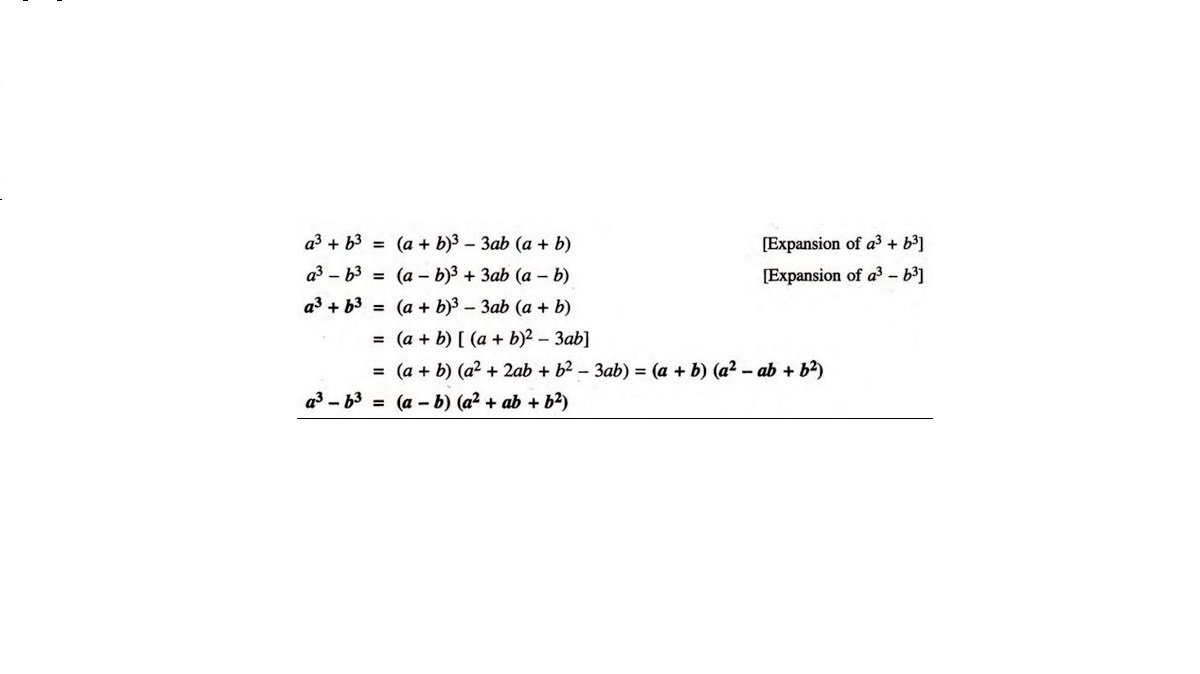
a³b³ Formula Proof- a3+b3, a3-b3 Formula Solution

The a^3+b^3 formula, a^3- b^3 formula Solutions are given below step by steps.

a^3-b^3 formula

check here a3 b3 Formula derivation with proof.

a³ – b³ = (a – b)(a² + ab + b²)

This can be proved by considering right hand side term.

(a – b)(a² + ab + b²) = a(a² + ab + b²) – b(a² + ab + b² )

=(a – b)(a² + ab + b²) = a³ + a²b + ab² – a²b – ab² – b³ [by taking the common factor (a-b) out]

=a³ + a²b – a²b + ab²– ab² – b³ [by bringing the like terms together and canceling the possible terms like a²b and ab² in the right hand side term]

= (a – b)(a² + ab + b²) = a³ – b³ = L.H.S

a^3+b^3 formula

The formula you’re referring to is most likely the sum of cubes formula:

a^3 + b^3 = (a + b) * (a^2 – ab + b^2)

To prove this formula, we can use algebraic manipulation. Start with the left-hand side of the equation:

a^3 + b^3

Now, let’s factor the sum of cubes on the left-hand side:

a^3 + b^3 = (a + b) * (a^2 – ab + b^2)

Now, let’s expand the right-hand side of the equation to see if we can obtain the left-hand side:

(a + b) * (a^2 – ab + b^2) = a * (a^2 – ab + b^2) + b * (a^2 – ab + b^2)

Distribute the terms within each parenthesis:

a * a^2 – a * ab + a * b^2 + b * a^2 – b * ab + b * b^2

Simplify each term:

a^3 – a^2b + ab^2 + a^2b – ab^2 + b^3

Notice that the terms “-a^2b + a^2b” and “-ab^2 + ab^2” cancel each other out:

a^3 + b^3

Voila! We have obtained the left-hand side of the equation, which proves the sum of cubes formula:

a^3 + b^3 = (a + b) * (a^2 – ab + b^2)

This formula is a useful tool in algebraic manipulations involving cubic expressions.

a3 b3 Formula Solutions And Sample Examples

Some a3 b3 Formula-based sample questions and examples are given below.

Problem: Factorize 27x³ – 64y³
Solution: We can write 27x³ – 64y³ as (3x)³ – (4y)³.

Therefore, by using the formula:

a³ – b³ = (a – b) (a² + ab + b²),

we get the factors as:

(3x – 4y) (9x² + 16y² + 12xy)

Problem: Factorize x³ + 8

Solution: The given equation x³ + 8 can be written as:

x³ + 2³. therefore, it is of the form,

a³ + b³.

Hence, by using the formula:

a³ + b³ = (a + b) (a² – ab + b²)

we get the factors as:

(x + 2) (x² – x . 2 + 2²)

= (x + 2) ( x² – 2x +4)

Problem: Find 8³ – 3³
Solution:  By using the formula:

a³ – b³ = (a – b) (a² + ab + b²),

we get the solution as:

(5) × (8² + 9 + 24) = 485

Problem: Factorize (2x + y)³ – (x + 2y)³
Solution: As this given equation is of the form a³ – b³,

Therefore, by using the formula:

a³ – b³ = (a – b) (a² + ab + b²),

we get the factors as:

(2x + y – x – 2y) [(2x + y)² + (x + 2y)² + (2x + y) (x + 2y)]
= (x – y) (7x² + 7y² + 13xy)

Related Post:

Sharing is caring!