Area of Rectangle, Formula, Definition, Derivation, Examples

Imagine you want to fill a rectangular park with artificial grass. But Do you know how much grass you need to cover the whole park? To determine the quantity of the grass first, you must know the area of that rectangular park. So, understanding the Area of the Rectangle Formula is necessary not only in maths but also in daily life. In this article, we are going to learn about the Area of the Rectangle Formula, its derivation with some solved Examples,

What is Area of Rectangle

In geometry, the Area of rectangle defines as the region or area covered by the rectangle in a two-dimensional plane. Before understanding the area of the Rectangle formula in Detail, we must get a general overall view of an Rectangle. A rectangle is a sort of quadrilateral, a two-dimensional object with four sides and four vertices. The rectangle’s four angles are all right angles or equal to 90 degrees. The rectangle’s opposite sides are equal and parallel to one other.

What is Area of Rectangle Formula

The area of rectangle os the is the space enclosed by the rectangle’s boundaries. The area of rectangle can also be defined as the number of unit squares that can fit inside it and is expressed in terms of the ‘number of’ square units.  So, We can easily determine the area of a rectangle which is the product of its length multiplied by its breadth. This can be determined using the area of rectangle formula and several approaches based on the dimensions provided.

Area of Rectangle Formula

The area of rectangle can be calculated by using the area of rectangle formula to determine how much space these things take up. Once the length and breadth of a rectangle are determined, the area is calculated. The area of a rectangle can be calculated by multiplying its length and width. As a result, the formula for the area of a rectangle whose length and width are ‘l’ and ‘w’ are as follows.

The area of rectangle Formula = (Length × Width)

How to Calculate Area of Rectangle?

Go through below mentioned few easy steps to calculate the area of a rectangle using the area of rectangle formula:

Step 1: Take note of the length and width (breadth) dimensions of the given rectangle.
Step 2: Multiply the length and width values of the rectangle to obtain the area of rectangle.
Step 3: Provide the solution in square units.

Unit of Area of Rectangle

The area of a rectangle is calculated by multiplying its length by its width (breadth). Square units are used to express the area of a rectangle. We all know that length is always measured and stated in units such as centimeters, inches, and so on. Because the area of a rectangle is calculated by multiplying its length and width, the area of the rectangle will be in square units. The units used to express the area of rectangles is always square units such as square centimeters, square inches, square feet, etc.

Area of Rectangle Using Diagonal

In some geometric problems, the length of the rectangle is not given. If the diagonal and one side of a rectangle are specified, the area can be computed. A rectangle’s diagonal is the straight line connecting the rectangle’s opposite vertices. A rectangle has two diagonals that are both the same length. Lets’s understand how to find the area of rectangle using the diagonal.

Using Pythagoras’ theorem, we can find the value of the missing side and then the area. The diagonal of a rectangle is determined using the following formula:

(Diagonal)² = (Length)²  + (Width)² 

If the Length of the given rectanagle is not given,

(Length)² = (Diagonal)²– (Width)²
(Length) =√ (Diagonal²– Width)²

Or,

If the Widh of the given rectangle is not given,

(Width)² = (Diagonal)² – (Length)²

(Width) =√ (Diagonal²– Length))²

Now, using the area of rectangle formula we can easily find out the area of the given rectangle.

Area of rectangle  = Length × Width

Area of Rectangle Formula Derivation

Now, The question arises of how the area of rectangle formula derives, Let us understand the derivatization of the area of rectangle formula. suppose ABCD is a rectangle with a diagonal AC. The diagonal AC divides the rectangle ABCD into two congruent triangles as (ABC and ADC) , As per the below diagram, We can conclude that the area of rectangle is the sum of the areas of these two triangles.

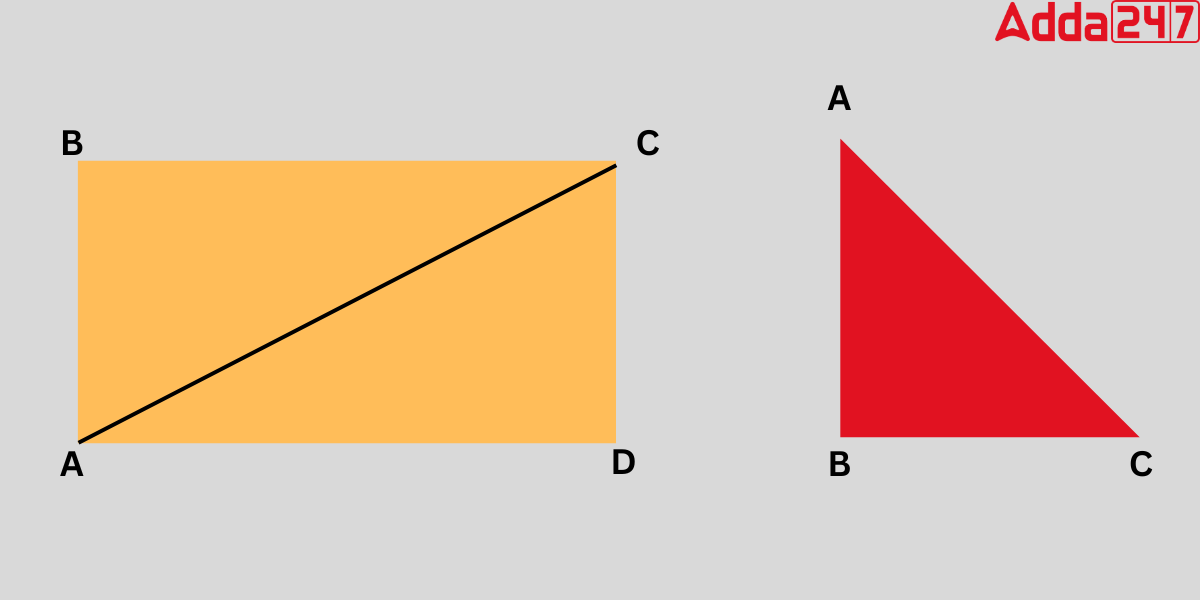
Derivation of the area of a rectangle formula
Area of Rectangle ABCD = Sum of the area of two congruent triangle
Area of rectangle ABCD = Area of ∆ABC + Area of ∆ADC
Or, Rectangle ABCD = 2 × Area of Triangle ABC
Or, Area of Rectangle  = 2 × (1/2 × Base × Height)
or, Area of ABCD = 2 × 1/2 × AB × BC
or, Area of Rectangle ABCD = AB × BC
or, As a result, Area of Rectangle = Length x Width. [proved]

Area of Rectangle Formula Examples

Example 1: The length and width of a rectangular park are 10 km yards and 6 yards. Find the total area of the parks

Solution:

As per the Given data, the Length of the park, l = 10 Km, and the width of the Park, w = 8 Km/.
Earlier, We know that the area of the rectangle formula is,
Area of rectangle = length × width
Or, The Area of the given rectangular park is = 10 × 8 Square km.= 80 km²
Therefore, the area rectangular park is 80 square km.

Example 2: Suppose a rectangle has a diagonal of 25 units, and a width of 3 units, Find the area of  rectangle.

Solution: Earlier we came to know that To find the Area of Rectangle we need its Length and Width.

We can find out the Length of the given rectangle using the area of rectangle formula,

(Length)² = (Diagonal)²– (Width)²
(Length) =√ (Diagonal²– Width)²

(Length) =⎷[(5)² – (3)²]

(Length) = ⎷[25- 9]

(Length) = = ⎷(16) = 4 units.

Area of the given rectangle = Length × Width = 4× 3 =12 square units.

Sharing is caring!