Bernoulli’s Theorem- Statement, Equation, Derivation, Applications

Bernoulli’s Theorem: Bernoulli’s Theorem, also known as Bernoulli’s principle, is an important theorem related to the movement of fluids. The formula is named after the famous Swiss Mathematician and Physicist Daniel Bernoulli. He postulated this theorem in 1738. This theorem provides a deep insight into fluid dynamics and explains the nature of the fluid while moving. It is applicable for a streamlined or steady flow. In this article, we will go through various aspects of this principle and will cover its formula, derivation, and applications.

Bernoulli’s Theorem

Bernoulli’s theorem is an important theorem for moving fluids that relates their pressure and velocity. The theorem states that the total amount of pressure energy, kinetic energy, and potential energy per unit volume is constant along any streamline flow of fluid. In other words, the combined mechanical energy of a moving fluid, which consists of the gravitational potential energy of elevation, the energy related to fluid pressure, and the kinetic energy of fluid motion, remains constant. This theorem is basically based on the conservation of energy principle. It must be noted that fluids comprise both liquids and gases. Using this theorem, it can be said that the pressure of a fluid is inversely related to its velocity, i.e.,

P ∝ 1/V

where P = pressure of the fluid

V = velocity of the fluid

Bernoulli’s Theorem Equation

The statement of the Bernoulli’s theorem stated above can be converted into mathematical expression. This is also known as the Bernoulli’s Theorem equation or Bernoulli’s Principle Formula.

The equation of Bernoulli’s Theorem is given by:

P + ρgh + (1/2)ρv² = constant

where, P = pressure

ρ = density of the fluid

v = velocity of the fluid

h = height of the fluid container

g = acceleration due to gravity

It is also known as Bernoulli’s equation.

Bernoulli’s Theorem Derivation

We can find out the formula written above for the Bernoulli’s principle. Let us derive this formula using a diagram.

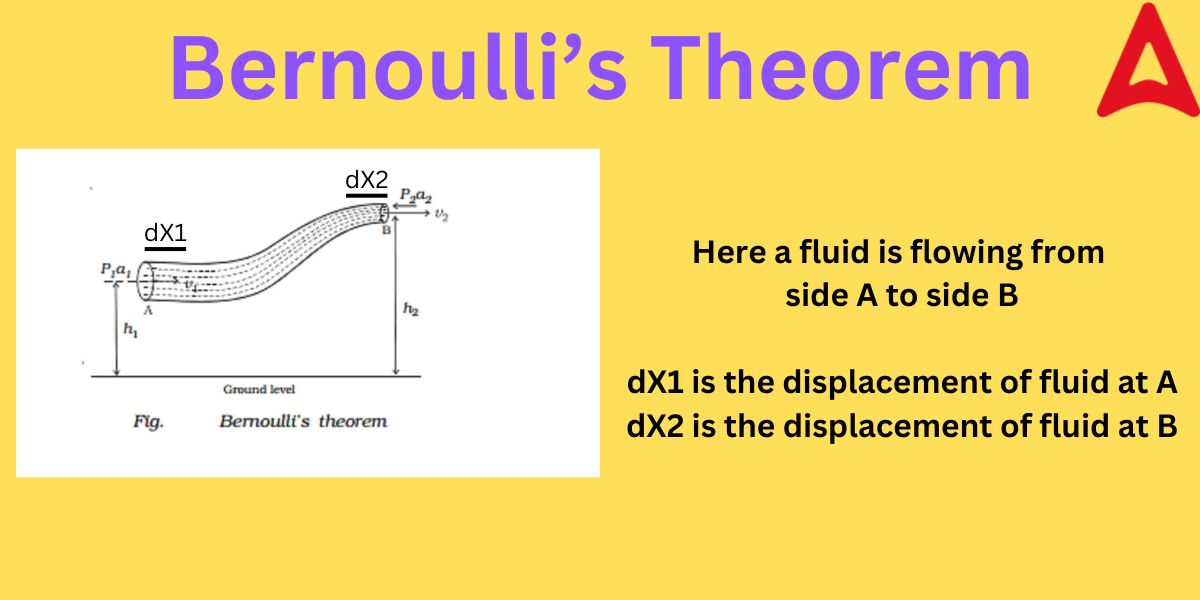
In the above figure we can see that a fluid is flowing from Side A to side B

The height as side A is h1 while the height of the fluid at Side B is h2

The cross section area at A = a1

Cross section area at B = a2

The pressure of fluid at side A = P1

pressure of the fluid at side B = P2

Velocity of the fluid at side A = V1

Velocity of the fluid at side B = V2

As we know that fluid flows from high pressure to low pressure, so P1 > P2

Let us assume that during a certain period of time, the fluid displaced at side A be dX1 while the displacement of the fluid be dX2 at side B so that the same amount of fluid volume is passed.

Then a small amount of work done (dw) on the fluid will be given by = F1.dX1 – F2.dX2

where F1 is the force applied at side A and F2 is the force applied at side B

as F = P.A

where P = pressure and A = area

so, dw = P1.a1.dX1 – P2.a2.dX2

as area and length = volume, so, a.dX = dv

dw = P1.dv – P2.dv

or, dw = dv (P1-P2)

The total energy of the given fluid system will be equal to the change in the potential energy and kinetic energy of the fluid.

i.e., dw = du + dk (because we assume that no viscous force is present) —–(i)

where du = change in potential energy and dk = change in kinetic energy

dk = (1/2)m1.(V1)² – (1/2)m2.(V2)²

as we go with the assumption that the density of the fluid remains constant at all the points

as mass = density x volume

so, dk = (1/2)ρ.dv.(V1)² – (1/2)ρ.dv.(V2)²

dk = (1/2)ρ.dv(V1² – V2²)

du = m2.g.h2 – m1.g.h1

du = ρ.dv.g.h2 – ρ.dv.g.h1

or, du = ρ.dv.g(h2-h1)

putting the values of dw, du, and dk in equation (i), we get

dv (P1-P2) = ρ.dv.g(h2-h1) + (1/2)ρ.dv(V1² – V2²)

cancelling out dv from each term, we get

(P1-P2) = ρ.g(h2-h1) + (1/2)ρ(V1² – V2²)

on rearranging the above terms, we get

P1 + ρ.gh1 + (1/2)ρV1² = P2 + ρ.gh2 + (1/2)ρV2²

as we can see the total energy is conserved at both points

Hence, P + ρgh + (1/2)ρv² = constant

Bernoulli’s Theorem and Conservation of Energy

It is important to note that the Bernoulli’s theorem obeys the principle of conservation of energy. In other words, we can use the principle of energy conservation to obtain the equation for the Bernoulli’s equation. As we can observe from the above derivation that the net work done by the fluid results from its change of potential energy (due to height difference) and change in kinetic energy (due to velocity). If we take the viscosity of the fluid into account, then, the equation will also have to include the thermal energy into account. Simply put, Bernoulli’s theorem obeys the energy conservation rule, i.e.,

total energy of a fluid = constant at all points in its flow

Bernoulli’s Theorem Equation PDF

As this theorem is one of the most asked topics in examination, so it is important for students to learn this concept thoroughly. Below we have provided the PDF for the Bernoulli’s principle equations in different cases. These equations will help students in solving different types of problems.

Bernoulli’s Theorem Equations

Bernoulli’s Theorem Applications

There are many applications of the Bernoulli’s theorem in the real world. This theorem is widely used in many disciplines, including cardiovascular physiology and aviation. Some of the applications of Bernoulli’s theorem are stated below.

• Finding Pressure Drops in Pipes: The pressure drop via a pipe can be calculated using this theorem. The friction between the fluid and the pipe walls is what results in the pressure drop.
• Aerodynamics: The lift produced by airplane wings is explained by Bernoulli’s theorem. The design of the wings makes the upper surface of the wing move air more quickly than the bottom surface. As a result, there is a pressure difference between the wing’s top and bottom surfaces, which produces lift.
• Cardiovascular System: Hemodynamics uses this Theorem to analyze blood flow in the circulatory system. It adds to the explanation of events like the Bernoulli effect, in which decreased pressure causes heart valves to close.
• Venturi meter: It is a tool that gauges how quickly liquid moves through pipes and is based on Bernoulli’s principle.
• Hydraulic Engineering: Engineers employ Bernoulli’s principle to create effective water purification systems, pipelines, and dams. The theorem helps these systems’ fluid flow and pressure distribution be optimized.

Bernoulli’s Theorem Examples

Some of the solved questions related to Bernoulli’s principle is given below so that students can have a proper understanding of this topic.

Example 1: A horizontal pipe filled with water flowing from point A to B. Find the pressure at a different place when the pressure at point A is 500 Pa, given the velocity of water is 2 m/s at A and 1m/s at B, and the height difference is insignificant.

Solution: As the height is constant, so the potential energy component will be nil

Hence, the Bernoulli’s theorem equation for such case becomes

P1 + (1/2)ρV1² = P2 + (1/2)ρV2²

given, P1 = 500 Pa

V1 = 2m/s

V2 = 1 m/s

as ρ (density of water) = 1000 kg/m³

putting these values in the standard equation

500 + (1/2) x 1000 x 2² = P2 + (1/2) x 1000 x 1²

500 + 2000 = P2 + 500

P2 = 2000 Pa

Example 2: What will be the pressure exerted by a static fluid at a point 10 meter above the ground if the pressure at at point 5 m above the ground is 25 Pa. Take the density of the fluid to be 500 kg/m³ and g as 10.

Solution: As the fluid is static so the velocity will be 0

Hence, the kinetic energy component will be nil

Using the modified equation of Bernoulli’s principle

P1 + ρ.gh1 = P2 + ρ.gh2

given, P1 = 25 Pa

ρ = 500 kg/m³

h1 = 5m

h2 = 10m

g = 10

substituting these values in the standard equation

25 + 500 x 10 x 5 = P2 + 500 x 10 x 10

25 + 25000 = P2 + 5000

P2 = 2025 Pa

Example 3: What will be the total energy of an ideal fluid at the height 100 meter, if its total energy is 500 Joule at the height 50 meter?

Solution: As we have been given total energy at height 50 m = 500 Joule

As the Bernoulli’s theorem is based on the conservation of energy

Hence, total energy = constant in an ideal fluid

Hence the total energy will remain the same

So, total energy at the height 100 m = 500 Joule

Sharing is caring!