Complementary Angles: Meaning, Types, Properties, Solved Examples

Complementary Angles are those angles whose sum is equal to 90°. That is, if the sum of two angles produces a right angle, then they are said to be complementary to each other. This property is related to the addition of angles. These angles form a right angle when added together. Both angles are said to be the complement of each other. Let us say that one angle is 40° and the other angle is 50°. when added together they give a right angle, i.e., 90°. So these two angles are called complementary in nature. In this case, 40° is called the complement of the 50° and vice versa.

Complementary Angles

Complementary angles are based on the concept of the angle sum properties of given angles. That means, whether the two given angles are complementary or not is decided by the measurement of their sum. If the sum equals exactly 90° then they are said to be complementary otherwise they are said to be non-complementary. It is important to understand that these angles are always in pair. The two angles may exist in the same figure or can be present alone too.

Complementary Angles Introduction

These Angles hold a special position in the geometrical world due to its unique nature of producing a right angle when added together. Angles are basic building blocks of geometry that are essential for defining shapes, calculating rotations, and resolving practical issues. These special angles stand out among the other sorts of angles because of their distinctive relationship with the core idea of a 90-degree angle.

Complementary Angles Definition

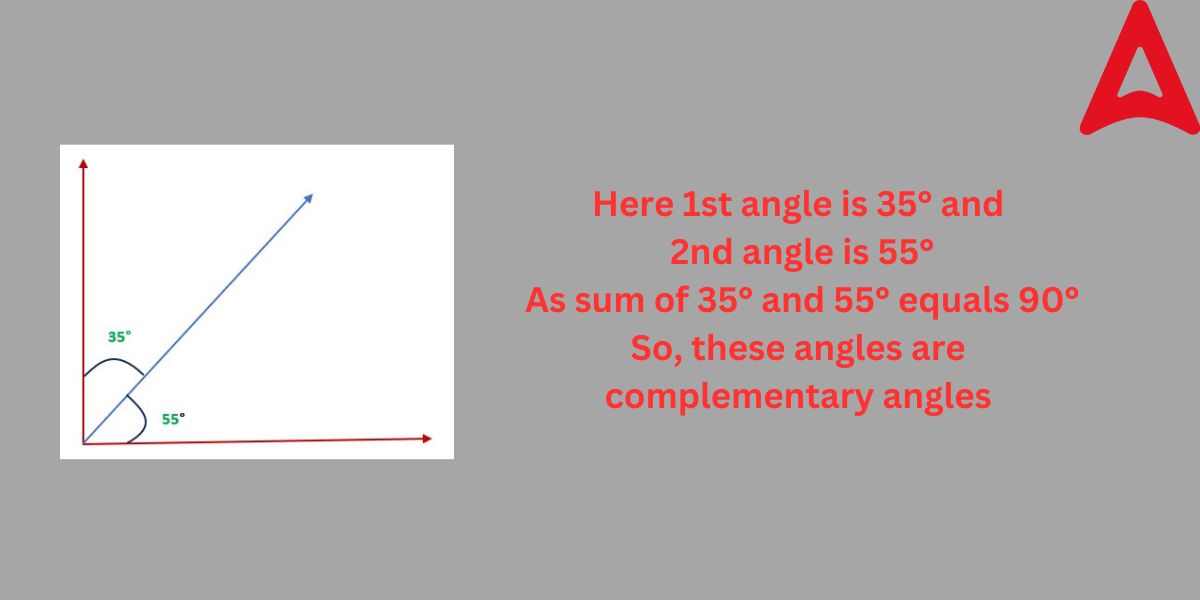
As per the formal mathematical definition, the pair of angles is said to be complementary if the addition of the two angles equals the measurement of a right angle. If the total of two angles is exactly 90°, they are said to be complementary angles. In other terms, a right angle (90 degrees) is formed when two angles that are complementary to each other are added together. If the sum of angles 1 and 2 equals 90 degrees (i.e., 1st angle + 2nd angle = 90°), then the angles are complementary and are referred to as one another’s complements. As shown in the figure below, one angle is 35° while the other angle is 55°.

1st angle = 35°

2nd angle = 55°

=> 35° + 55° = 90°

As 1st angle + 2nd angle = 90°

Therefore, the given angles are complementary in nature.

Here, 55° is the complement of 35° and

35° is the complement of 55°.

Complementary Angles Formula

The formula for complementary angles are quite simple. The complementary angle formula is shown below.

Let us consider two angles: A and B

If angle A + angle B = 90°

Then angle A and angle B are said to be complementary.

Complementary Angles Types

There are basically two types of these special angles.

1. Adjacent Complementary Angles: If two angles have a common vertex and a common arm, i.e., are adjacent to each other and their sum equals 90°, then they fall in this category of angles that are complementary.
2. Non-adjacent Complementary Angles: If the sum of two angles are equal to 90° and they are not adjacent to each other, then they fall in this category of angles that are complementary.

Complementary Angles in Trigonometry

These angles play an important role in trigonometry. As we know that trigonometry is centered around the concept of right angle. As in these angles, if one angle is A° then other angle must be (90°-A°). So we can use this unique concept in trigonometry, where one ratio is complementary to another trigonometric ratio by 90°. The same is shown below.

cos (90° – X) = sin X and vice versa

cot (90° – X) = tan X and vice versa

cosec (90° – X) = sec X and vice versa

As a result, you can observe that if the angles complement one another, the trigonometric ratio of the angles changes.

Complementary Angles Properties

These angles shows many unique properties. These properties are useful in solving many complex academic and real-life problems. By learning the properties given below, you will be able to solve many different types of problems related to these angles easily. Some of the unique properties related to these angles are herein:

• They always occur in pairs.
• More than two angles cannot be complementary even if their sum equals 90°
• The sum of these angles always result in 90°
• Only acute angles can form complementary pairs
• Acute angles of a right-angled triangle are always complementary in nature
• The angles that are complementary are known as complement of one another

Complementary Angles Solved Examples

Some of the solved examples of problems based on this topic are given below. These solved examples will help students to master this concept in a more precise way.

Example 1: What will be the complement of 40°?

Solution: As we are given with the angle 40°

The complement of 40° = 90° – 40°

= 50°

So the complement of 40° is 50°.

Example 2: There are two angles that are complementary to one another. The measure of one of the angles is given to be 30°. What will be the other angle’s measurement?

Solution: Given 1st angle = 30°

As we know the sum of angles complementary to each other= 90°

i.e., 1st angle + 2nd angle = 90°

Let 2nd angle be x

So, 30° + x = 90°

x = 60°

Example 3: Find out the complement of 30°+3a.

Solution: As we know, to find the complement, we subtract the given value from 90°

i.e., the complement of 30°+3a = 90° – (30° + 3a)

So, the complement of 30°+3a = 60°-3a

Sharing is caring!