Cos 60 Value: Check Cos 60° ka Maan -

Table of Contents

Cosine is a fundamental trigonometric function, representing the ratio of the adjacent side to the hypotenuse in a right-angled triangle. One of the most commonly known values in trigonometry is cos 60°, equal to 0.5. This value is widely used in mathematics, physics, and engineering to simplify calculations and solve problems involving angles and distances. Understanding cos 60° is essential because it serves as a basis for many mathematical concepts and applications, from constructing angles to calculating forces in physics. This article explores the importance, derivation, and applications of cos 60° in various fields, showcasing its practical significance.

Cos 60 degree

The value of cos 60 degree is (0.5 in decimal form) or 1/2. Trigonometry is used in right angled triangles to calculate parameters such as the length, height, and angle of the triangle.

There are six types of trigonometric functions which we use primarily. They are

sine
cosine
tangent
cotangent
secant
cosecant

The standard angles of the trigonometric ratios are 0°, 30°, 45° 60°, 90°.

Read about value of root 2 and square root of 2.

Cos 60 degree value

Trigonometric functions such as sine and cosine are used in the theory of periodic functions. These periodic functions are used to describe the light and sound waves. It is also used in oceanography to calculate the height of tides in oceans. Apart from these applications, trigonometry is also used by astronauts, physicists, and engineers.

What is the value of cos 60 degree in fraction?

The value of cos 60° is (0.5 in decimal form).
cos 60-degree fraction value is= 1/2 .
You can also derive cos 60° value by having a look at the next section.

Value of Cos 60 degree

Follow the instructions given below to derive the value of cos 60°.

Consider a unit circle in the Cartesian plane. The plane can be divided into four quadrants. The cos 60° value takes place in the first quadrant.

90° – 30° = 60° (Equation 1)

sin (90° – a) = cos a (Trigonometric formula)

Now, we can write the value of cos 60° as,

sin (90° – 60°) = cos 60° (Using the trigonometric formula)

sin 30° = cos 60° (Equation 2)

Since the value of sin 30° is , by substituting the value of sin 30° in equation 2 we get,

= cos 60°

Hence the value of cos 60° is .

Alternatively,

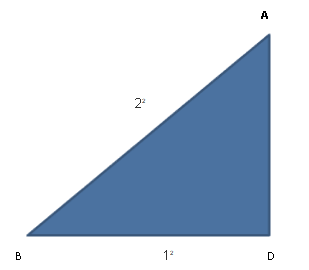
In right angled triangle ABD,

AB² = AD²+ BD²

2²= AD²+ 1²

AD² = 2²-1²

AD² = 4 – 1

AD² = 3

AD = √3

Hence,

sin 60° = AD/AB = √3/2

tan 60° =AD/BD =√3/1

cos 60°= 1/2

Read about table of 6.

Cos 60 degree Value from Trigonometry Table

Angle in Degrees	0°	30°	45°	60°	90°
Angle in Radians	0	π/6	π/4	π/3	π/2
sin	0	1/2	1/√2	√3/2	1
cos	1	√3/2	1/√2	1/2	0
tan	0	1/√3	1	√3	Not defined
cosec	Not defined	2	√2	2/√3	1
sec	1	2/√3	√2	2	Not defined
cot	Not defined	√3	1	1/√3	0