de Broglie Wavelength- Definition, Equation, Derivation, Examples

The de Broglie wavelength is one of the most interesting phenomena associated with quantum mechanics. This concept helps us comprehend the dual nature of a matter, i.e., a matter can show both particle-like properties and wave-like properties. Due to this, it forms an important component of the modern physics. This concept has great importance in both physics and chemistry. Due to its importance in the board and competitive exam, we have covered all its notes and provided students with a detailed notes’ PDF.

de Broglie Wavelength

de Broglie wavelength are basically the waves associated with the particle. So the de Broglie concept shows that a particle can also show the nature of waves. The de Broglie wavelength, a fundamental quantity that connects a wavelength with a particle, was first proposed by this idea, also known as wave-particle duality.  We do not perceive waves in the commonly observed objects since their wavelengths are so small and undetectable. However, one can observe the formation of the these wavelengths in the case of subatomic particles. This proposition shows that just like light (photons), other particles like electron and proton also exhibit wave properties.

Louis de Broglie made a ground-breaking claim that particles, which are typically conceived of as discrete particles, might also display wave-like properties early in the 20th century when the concepts of quantum mechanics began to take shape. It led to the naming of the concept after him, hence the name. This topic explains the duality nature of matters.

de Broglie Wavelength Definition

As per the formal definition, de Broglie wavelength is the wavelength that is connected to an object in relation to its momentum and mass. In other words, the wavelength associates with every matter. This wavelength shows us that, just like the light, every other moving particles form waves. These waves are known as de Broglie waves or matter waves (as they are associated with the matter). Louis de Broglie proved through his experiment that every matter exhibits particle and wave characteristics, also known as the dual nature. It is denoted by the symbol lambda (λ). The force of a particle is generally inversely related, i.e., inversely proportional to its wavelength.

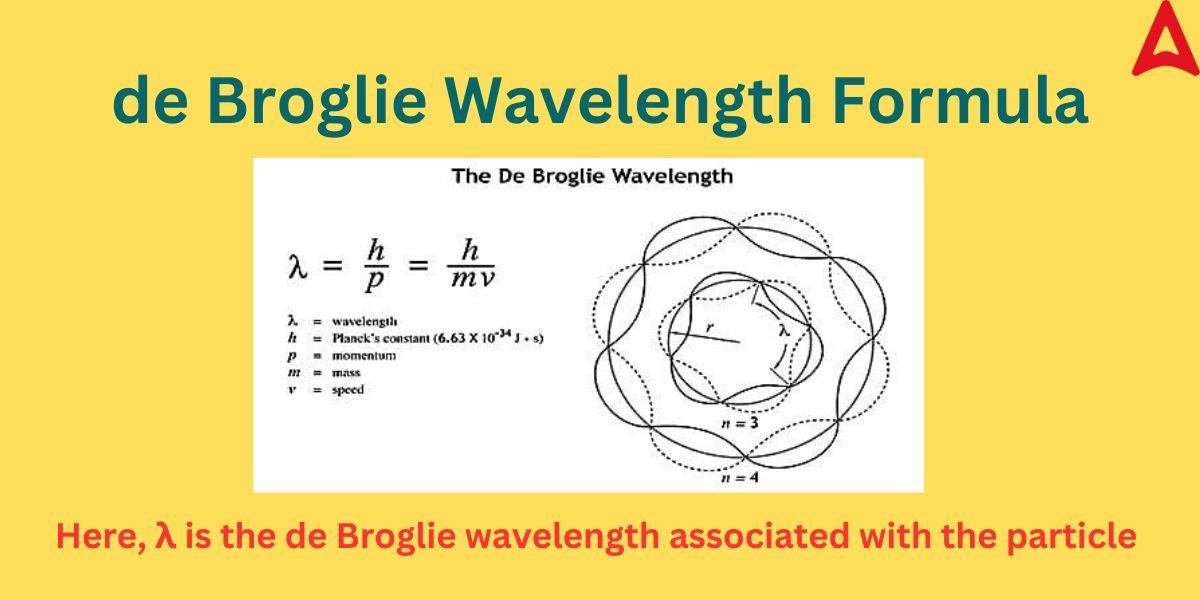
de Broglie Wavelength Formula

The de Broglie wavelength formula is used to derive the wavelength associated with various particles. This formula is also sometimes known as de Broglie wavelength equation. The Formula states that wave-particle duality is a fundamental property shared by radiation and matter, as opposed to being a peculiar property of light. This equation connects a wave or particle’s momentum to its wavelength.

The formula is expressed as following:

λ = h/mv

or λ = h/p

where λ = wavelength associated with particles

m = mass of the matter

v = velocity of the matter

h = Planck’s constant (6.63 x 10^-34 Js)

P = particle’s momentum = mv

As we know, momentum (p) is a particle property while wavelength (λ) is a wave property, so, this formula establishes the relation between the wave nature and particle nature of matter though the Planck’s constant.

de Broglie Wavelength of Electron

Just like any other matter, we can derive the wavelength of the electron using the formula mentioned above. The wavelength in electrons are created when it revolves around the nucleus of the atom and it exist in the form of loop. De Broglie waves can only exist as standing waves and fit uniformly around the closed loop that results from electrons revolving around atom nuclei in this scenario. As a result of this circumstance, the electrons in atoms orbit the nucleus in particular configurations or states that are referred to as stationary orbits. The wavelength associated with the electron is given by:

λ_e = h/m_ev

where λ_e = de Broglie wavelength of the electron

m_e = mass of the electron = 9.1 x 10^-31 kg
v = velocity of the electron

de Broglie Wavelength of Proton

Just like the electron, de Broglie can be calculated for the proton. Proton are positive charged elements situated in the nucleus of the atom. The wavelength associated with the proton is highly important in the study of nuclear reactions. The wavelength associated with the proton is given by:

λ_p = h/m_pv

where λ_p = de Broglie wavelength of the electron

m_p = mass of the electron = 1.67 x 10^-27 kg
v = velocity of the proton

de Broglie Wavelength Units

As the de Broglie wavelength is a length, so its unit is same as that of the length or light wavelength. The SI unit is meter but due to its very small size, it is often expressed in Nanometer (10^-9) or Angstrom units (10^-10). We can also derive its unit by using the basic concept of unit calculation.

de Broglie Wavelength Derivation

The matter wavelength formula (stated above) can be derived using mathematical concepts and some basic understanding. We can derive the formula for the de Broglie wavelength using Einstein’s mass-energy energy equivalence and the quantum energy concept. The derivation for the formula is given below.

As we know, each quantum of wave possess some discrete amount of energy which is given as:

E = hf —————-(i)

where, E = particle’s energy

h = Planck’s constant (6.63 x 10^-34 Js)

f = frequency

According to Einstein’s mass-energy theorem,

E = mc² —————- (ii)

where, E = particle’s energy

m = mass of the particle

c = speed of light

The De-Broglie hypothesis states that the behavior of Broglie’s particles and waves is analogous. He did this by equating the particle and wave energy relations. So, by equating equation (i) and equation (ii), we get:

mc² = hf

De Broglie substituted the speed of light “c” with the velocity of a real particle “v”, since particles don’t generally move at the speed of light. On doing so, we get:

mv² = hf  ————— (iii)

As we know, frequency of the wave (f) = velocity of the wave (v)/wavelength of the wave (λ)

on putting the value of f in equation (iii)

mv² = hv/λ

or, mv = h/λ

Hence, λ = h/mv

or, λ = h/p, where p = momentum of the particle

de Broglie Wavelength Kinetic Energy

We can relate the kinetic energy of a particle with its wavelength by using the de Broglie wavelength formula. Let us derive the relation between the kinetic energy and wavelength of a particle.

As we know, Kinetic Energy (K) = (mv²)/2 = p²/2m

or, 2mK = p²

p = √2mK

where, m = mass of the particle

v = velocity of the particle

p = momentum of the particle

As the wavelength formula for a particle is expressed as:

λ = h/mv or h/p

putting the value of p in the above equation, we get:

λ = h/√2mK

de Broglie Wavelength in terms of Temperature

The wavelength associated with matters can be defined in terms of temperature too. It is known as the thermal de Broglie wavelength. It is associated with gaseous particles. At the given temperature, the thermal de Broglie wavelength (λ_D) is roughly equal to the average wavelength of the gas particles in an ideal gas. The wavelength formula in terms of temperature is given by:

λ_D = h / √ 2 π m k_BT

where,

m = mass of a gas particle

h = Planck’s constant

T = temperature of the gas

k_B = Boltzmann constant

λ_D = thermal de Broglie wavelength of the gas particles

de Broglie Waves Notes

The notes on de Broglie waves will not only give students a head-start in their annual examination but also in competitive exams. De Broglie Waves notes will help students learn and revise all the concepts of dual nature of matter for board as well as competitive exams. These notes contains important formulas, tricks and solved questions to fully prepare students for competitive exams like NEET exam. By going through the notes and video explanation of the de Broglie waves, students will surely get a complete clarity on this concept. The notes given below as well as the video explanation given in the subsequent section is more than enough for this concept. Students then won’t have to look for any other resources for this topic.

de Broglie Wavelength Examples

The wavelength associated with matters is an important concept both for class 11 and class 12 physics and chemistry curriculum. Some of the solved questions of the de Broglie wavelength is given below for students. After going through these solved examples, students will have a better understanding of this topic.

Example 1: What will be the wavelength of the particle whose mass is 2 x 10^-30 kg and is moving with a speed of 5 x 10⁷ meter/second?

Solution: Given,

mass (m) of the particle = 2 x 10^-30 kg

velocity (v) of the particle = 5 x 10⁷ m/s

As the wavelength associated with the particle is given by the de Broglie formula

Hence, λ = h/mv

where, h = 6.63 x 10^-34 Js

λ = 6.63 x 10^-34/2 x 10^-30 x 5 x 10⁷

λ = 6.63 x 10^-34/10^-30x 10⁸

λ = 6.63 x10^-34/10^-22

λ = 6.63 x 10^-12

so the wavelength of the particle = 6.63 x 10^-12 meter

Example 2: What will be the wavelength of the electron accelerated with the kinetic energy of 10 KeV

Solution: We have been given the kinetic energy (K) of the proton = 10 KeV

First, we will change the kinetic energy into its SI unit value

so, K = 10 x 1000 x 1.6 x 10⁻¹⁹

K = 16 × 10⁻¹⁶ J

As we know, the relation between the wavelength and the kinetic energy of a particle is given by:

λ = h/√2mK ——- (i)

mass (m) of proton = 9.1 x 10^-31 kg

on substituting all the known value in equation (i), we get:

λ = 6.63 x10^-34/√2 x 9.1 x 10^-31 x 16 × 10⁻¹⁶ J

On solving, we get:

λ = 3.90 × 10⁻¹⁰ m

Example 3: What will be the de Broglie wavelength associated with the particle whose momentum is 10^-27 kg-m/s²?

Solution: As given in the problem,

momentum of the particle (p) = 10^-27 kg-m/s²

The wavelength associated with the particle is given by:

λ = h/p

or, λ = 6.63 x10^-34/10^-27

or, λ = 6.63 x10^-34+27

λ = 6.63 x10^-7

or, λ = 6.63 x10^-34+27

λ = 6.63 x 10^-7

or, λ = 663 x 10^-9

Hence, λ = 663 Nanometer

de Broglie Waves Notes for NEET

The de Broglie waves key notes from the NEET Physics curriculum are provided below in PDF format. By clicking on the PDF link, candidates getting ready for the NEET and other exams can access and download this detailed notes covering all the aspects associated with de Broglie waves. Both last-minute exam preparation and full-exam preparation can be done by going through this PDF. The de Broglie Waves essential notes contain every important topic and concept that is pertinent to the NEET UG exam. In addition to the notes, the PDF includes significant NEET multiple-choice questions (MCQs) to help students completely understand and practice this chapter.

de Broglie Waves Notes for NEET PDF

The detailed PDF notes on this concept created by the expert faculty for NEET at Adda 247 is given below for download.

de Broglie Waves Notes PDF for NEET

de Broglie Waves Video Explanation

The detailed video explanation on this chapter is given below. The video contains all the important theory for the NEET exam along with important PYQs.

Sharing is caring!