Differentiation of Sec x, Formula, and Examples

Differentiation of Sec x: Differentiation of trigonometric ratios is one of the most interesting topics in higher mathematics. One such differentiation of the trigonometric ratio is the differentiation of Sec x. Before discussing the differentiation of trigonometric ratios, let us first recall the concept of differentiation. It will help us understand this concept in a more intuitive manner.

What is Differentiation?

Differentiation is one of the fundamental concepts in calculus. A key idea in calculus called differentiation enables us to look at how quickly one quantity changes in relation to another. Differentiation is essential to comprehending and modelling dynamic processes, whether we’re trying to grasp how objects move, examine how diverse industries are growing, or solve optimization issues. In other words, the differentiation tells us the rate of change of a particular entity with respect to another quantity. It is the opposite function of integration, which is also one of the fundamental  concepts in calculus.

The differentiation is denoted by dy/dx or y’

where y is the entity being differentiated

Differentiation of Sec x

The differentiation of Sec x, also known as the derivative of Sec x, means the first order derivative of the trigonometric ratio of Sec x. The first order derivative means the value is differentiated with respect to another value only once. In the second order derivative, the value is differentiated twice, and so on. Sec x or Secant x is the inverse ratio of Cos x. In other words, Sec x = (1/Cos x). Apart from Sec x and Cos x, other trigonometric ratios are Sin x, Tan x , Cosec x, and Cot x. The differentiation of Sec x can de denoted by d(Sec x)/dx or (Sec x)’. Tan x comes in the formula of the differentiation of Sec x. so, we should know that Tan x is the ratio between Sin x and Cos x, i.e.,

Tan x = Sin x/Cos x

Differentiation of Sec x Formula

The derivative of Sec x will give us the first-order rate of change of Sec x with respect to x. The formula for the same is denoted below.

The differentiation of Sec x is given by:

d (Sec x)/dx = Sec x . Tan x

or (Sec x)’ = Sec x . Tan x

As you can observe that the derivative of Sec x gives us Sec x . Tan x. Let us now find the result of this formula (as given above) using different methods.

Differentiation of Sec x using First Principle

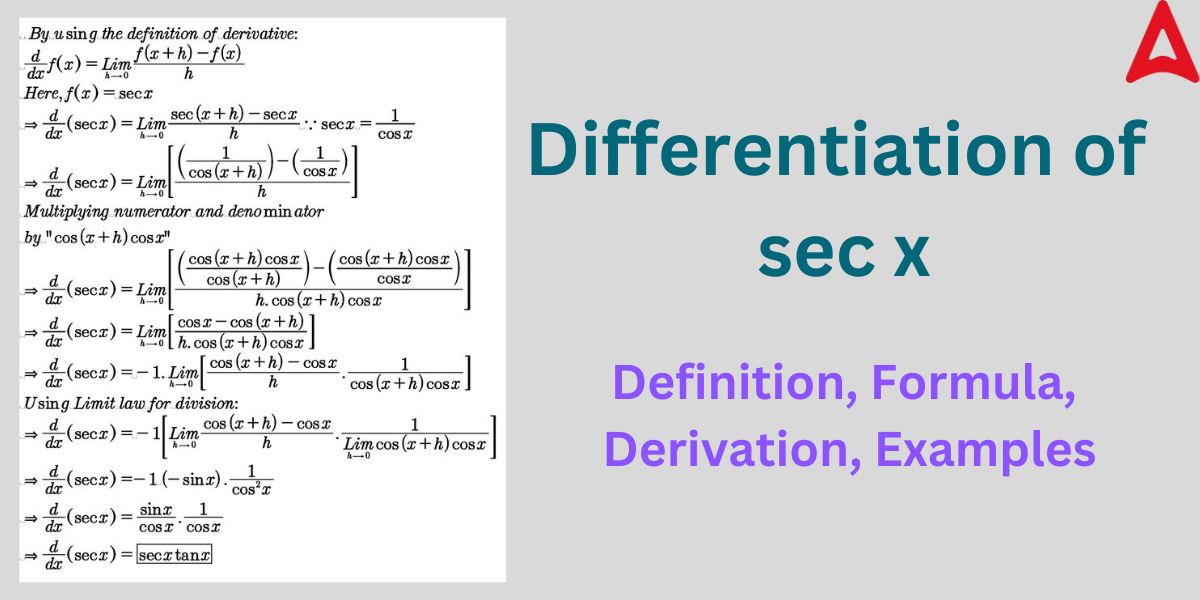
First Principle is one of the fundamental methods to find the differentiation of any function. It uses the concept of limits. The proof for the differentiation of Sec x using the First Principle is given below.

Using the first principle formula, the derivative of a function f(a) is,

f'(a) = limₕ→₀ [f(a + h) – f(a)] / h ………. (i)

where, f'(a) is the differentiation of a

h is a constant

In our case, f(a) is given to be Sec x

and f(a + h) = sec (x + h)

On substituting these values in (1),

f’ (x) = limₕ→₀ [sec (x + h) – sec x]/h

as Sec x = 1/Cos x

=> limₕ→₀ 1/h [1/(cos (x + h) – 1/cos x)]

=> limₕ→₀ 1/h [cos x – cos(x + h)] / [cos x cos(x + h)]

Taking the non-limit term cos x outside, we get

=> (1/cos x) limₕ→₀ 1/h [cos x – cos(x + h)] /[cos(x + h)]

Using the formula cos C – cos D = -2 sin (C+D)/2 sin (C-D)/2, we get

f'(x) = 1/cos x limₕ→₀ 1/h [- 2 sin (x + x + h)/2 sin (x – x – h)/2] / [cos(x + h)]

= 1/cos x limₕ→₀ 1/h [- 2 sin (2x + h)/2 sin (- h)/2] / [cos(x + h)]

Multiplying and dividing the above equation by h/2, we obtain

= 1/cos x limₕ→₀ (1/h) (h/2) [- 2 sin (2x + h)/2 sin (- h/2) / (h/2)] / [cos(x + h)]

When we put h → 0, we get h/2 → 0.

Hence, f'(x) = 1/cos x limₕ/₂→₀ sin (h/2) / (h/2). limₕ→₀ (sin(2x + h)/2)/cos(x + h)

As we know by the rule of the first principle limₓ→₀ (sin x) / x = 1.

So, f'(x) = 1/cos x. 1. sin x/cos x

We know that 1/cos x = sec x and sin x/cos x = tan x. So

f'(x) = sec x · tan x

Hence proved.

Differentiation of Sec x using Chain Rule

Chain rule is another way of finding the differentiation. We can calculate the differentiation of Sec x in the following way:

Here, f(x) = Sec x = 1/cos x

f(x) = 1/cos x = (cos x)^-1

By power rule and chain rule, we obtain

f'(x) = (-1) (cos x)^-2 d/dx(cos x)

By using the property of exponents, a^-m = 1/a^m, and d/dx(cos x) = – sin x, we obtain

f'(x) = -1/cos²x · (- sin x)

=> (sin x) / cos²x

= 1/cos x · (sin x)/(cos x)

as sin x/cos x = tan x and 1/cos x = sec x

f'(x) = sec x · tan x

Hence proved.

Differentiation of Sec x using Quotient Rule

The quotient rule is one of the basic rules of differentiation used in the case when two quantities are in division. The division rule states that if two quantities “x” and “y” are in division and we have to find its differentiation, then the differentiation of this equation using the quotient rule will be given by:

(x/y)’ = [x’y – y’x]/y²

using this rule, we can find the differentiation of Sec x as follows:

given f(x) = Sec x = 1/cos x

here, x = 1 and y = cos x

so applying the quotient rule stated above

f'(x) = [1′. cosx – 1. (cos x)’]/(cos x)²

as we know (cos x)’ = d(cos x)/dx = – sin x

and a’ = d(a)/dx = 0, where a is any constant

so, f'(x) = [0. cosx – 1. (- sin x)]/(cos x)²

f'(x) = sin x/(cos x)²

=> (1/cos x) . (sin x/cos x)

f'(x) = sec x . tan x

Hence proved

Differentiation of Sec x Solved Examples

After knowing the differentiation of sec x, we can now solve the problems related to sec x derivative. The following questions involve differentiation problems on some other form of sec x like differentiation of sec² x, differentiation of sec x . tan x, and differentiation of sec^-1 x. These examples will help you understand how to use the differentiation of sec x in a better way.

Example 1: Find the Differentiation of Sec² x

Solution: given f(x) = sec²x

f'(x) = (sec²x)’

using the differentiation rule of (xⁿ) = n (x^n-1), and chain rule, we get:

2 . sec x . (sec x)’

2. sec x. sec x. tan x

or, 2. sec²x. tan x

Hence, (sec²x)’ =2. sec²x. tan x

Example 2: Differentiate sec^-1 x.

Solution: given f(x) = sec^-1 x

i.e., we have to find the differentiation of sec^-1 x

so, f'(x) = (sec^-1 x)’ = d(sec^-1 x)/dx

There is a straight-forward formula for the sec^-1 x as per the rule for the differentiation of the inverse trigonometric ratios, which is given by

f'(x) = 1/[ |x|√x² – 1], where x ≠ 0, ±1

Example 3: What will be the first-order derivative of sec x . tan x

solution: We have been given f(x) = sec x . tan x

we can find the value of this function using the product rule property stated below:

(x.y)’ = x’y + xy’

where x and y are two quantities in multiplication

applying this rule on f(x)

f'(x) = (sec x . tan x)’

here, x = sec x and y = tan x

so, f'(x) = (sec x)’ . tan x + sec x . (tan x)’

using the value of differentiation of sec x = sec x. tan x and tan x = sec²x

=> sec x. tan x. tan x + sec x. sec²x

=> sec x . tan² x + sec³ x

Hence, f'(x) = sec x (tan² x + sec²x)

Sharing is caring!