Table of Contents
Candidates who are studying Mathematics whether for the school exam or the competitive exam should know about the divisibility rule of basic numbers. The divisibility rule is also known as the divisibility test. The divisibility rule of 11 is quite easy to understand and remember if you study it from the right resource.
To provide you with the correct explanation of the 11 divisibility rule, we have prepared this article. We bet you that after reading this post, you will be able to know if any number is divisible by 11 or not with ease.
Divisibility Rule of 11
11 is the smallest two digit odd number and well as prime number. Because being a prime number, students often face problem while dividing a number by 11. After learning the divisibility rule of 11, you will be able to tell if any number is divisible by 11 or not without the need for any mathematical calculation. The rule of divisibility for 11 outlines the criteria for determining if a number is divisible by 11 without any remainder.
For instance, the number 352 can be evenly divided by 11 with a remainder of 0.
11 Divisibility Rule
The 11 divisibility rule allows a person to determine if a whole number is divisible by 11. This specific rule declares that if the difference between the sum of digits at odd positions and the sum of digits at even positions in a number is either 0 or 11, then the number is divisible by 11. In subtraction, always subtract the larger value from the smaller value. The simplest and quickest method to determine if a number is divisible by 11 is by applying the divisibility rule of 11.
Rule for Divisibility of 11
The divisibility rule of 11 is given below.
According to the rule of divisibility by 11, if the total of the digits in odd positions subtracted from the total of the digits in even positions is either 0 or a multiple of 11, then the number in question can also be divided by 11. Always use the positive value of subtraction and forget the negative sign or you can make sure to subtract the larger sum from the smaller sum.
Step by Step Process for Divisibility Rule of 11
Listed below are the instructions that can assist you in determining if a number is divisible by 11 using the divisibility rule of 11:
- Begin with either the furthest right or furthest left digit of the provided number.
- Determine the total of the digits located in the odd positions in the provided number.
- Determine the total of the digits located in the even positions within the provided number.
- Next, deduct the lesser sum from the greater sum obtained in steps 2 and 3.
- If the resulting difference is either 0 or 11 or multiples of 11, then the number in question will be evenly divisible by 11 with no remainder.
Divisibility Test of 11 With Examples
Let us apply the above mentioned rule and step-wise process to test the divisibility rule for 11.
Example 1: Here we are applying the divisibility test of 11 on the number 65678932
Step 1: We will begin with the rightmost digit, i.e., 2
Step 2: Determining the sum of digits located at odd positions- 2 + 9 + 7 + 5 = 23
Step 3: Determining the sum of digits located on even positions- 3 + 8 + 6 + 6 = 23
Step 4: Finding the difference of the tow sum: 23 – 23 = 0
Step 5: A the difference of both these sum are 0, hence, the number 65678932 is divisible by 11.
Example 2: Applying divisibility test of 11 on the number 3784
Step 1: We will begin with the leftmost digit, i.e., 3
Step 2: Determining the sum of digits located at odd positions- 3 + 8 = 11
Step 3: Determining the sum of digits located on even positions- 7 + 4 = 11
Step 4: Finding the difference of the tow sum: 11 – 11 = 0
Step 5: A the difference of both these sum are 0, hence, the number 3784 is divisible by 11.
11 Divisibility Test Practice Questions
Determine whether the following numbers are divisible by 11 or not with the help of divisibility rule.
- 86416
- 9780
- 53469
- 86325
- 764852



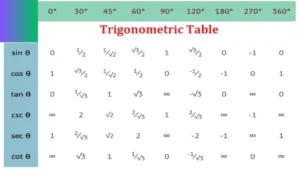 Trigonometry Table- Learn Sin Cos Tan Ta...
Trigonometry Table- Learn Sin Cos Tan Ta...
 Fraction to Percentage Table 1 to 20, Ch...
Fraction to Percentage Table 1 to 20, Ch...
 Divisibility Rule of 7: 7 Se Vibhajita K...
Divisibility Rule of 7: 7 Se Vibhajita K...






