Table of Contents
The Council for the Indian School Certificate Examinations (CISCE) has published the ICSE Class 10 Mathematics Specimen Paper 2025 on its official website. Candidates planning to take the ICSE Board exams should review the ICSE Class 10 Maths Specimen Paper 2024-25 to understand the test pattern and format of questions recommended by CISCE. We have shared the Section wise ICSE Class 10 Mathematics Specimen Paper 2025 along with PDF in this post,
ICSE Class 10 Maths Specimen Paper 2025
Maths is regarded as one of the most challenging subjects among pupils. With enough practice, one can easily pass the maths exam with a high score. The Best source for practice is the ICSE Class 10 Maths Specimen paper, which must be referred to understand the exam structure and marking scheme for the upcoming ICSE Class 10 Maths Board Exam 2025. ICSE Specimen paper of Class 10 Maths can provide insight into typical question patterns and topic distribution. This enables students to prioritize their study efforts based on the importance of certain sections.
Download ICSE Class 10 Maths Syllabus PDF
ICSE Class 10 Maths Specimen Paper 2024-25 Pattern
The format of ICSE Class 10 Maths specimen paper 2024-25 often resembles that of the actual Class 10 Matht est. Analysis of them allows students to become acquainted with the structure, question types, and time constraints. Check out the new sample paper for the CISCE Board Exam for more information about the ICSE Class 10 Maths Exam Pattern.
- The Class 10 Maths Exam carries 100 marks and is divided into two parts: the exam (80 marks) and the internal assessment (20 marks).
- The ICSE Class 10 Maths Exam will include both objective and subjective questions.
- The total number of marks for the theory Maths exam is 80, and students will have 2.5 hours to complete the exam.
- The question paper will be broken into two sections:
Section A: 40 marks, required short answer questions
Section B: 40 marks, 7 questions, any 4 to be answered
| Important Instructions |
|
ICSE Class 10 Maths Specimen Paper 2025 PDF Download
ICSE Class 10 Maths Specimen Paper 2025 PDF with Solutions is now available for download on the official website of the Council for the Indian School Certificate Examinations (CISCE) at cisce.org. For the convenience of the students here, we have provided the direct link to download the Council for the ICSE Class 10 Mathematics Specimen Paper Pdf.
Download ICSE Class 10 Mathematics Specimen Paper PDF
ICSE Class 10 Mathematics Specimen Paper 2025 Section A
SECTION A
(Attempt all questions from this Section.)
Question 1: Choose the correct answers to the questions from the given options. [15]
(Do not copy the question, write the correct answers only.)
(i) 𝐼𝑓 𝐴 = [−1 2] 𝑎𝑛𝑑 𝐵 =
Which of the following operation is possible?
(a) A – B
(b) A + B
(c) AB
(d) BA
(ii) If 𝑥² + 𝑘𝑥 + 6 = (𝑥 − 2 )(𝑥 − 3) for all values of x, then the value of k is:
(a) – 5
(b) – 3
(c) – 2
(d) 5
(iii) A retailer purchased an item for ₹1500 from a wholesaler and sold it to a customer at 10% profit. The sales are intra-state and the rate of GST is 10%. The amount of GST paid by the customer:
(a) ₹15
(b) ₹30
(c) ₹150
(d) ₹165
(iv) If the roots of equation 𝑥² − 6𝑥 + 𝑘 = 0 are real and distinct, then value of k is:
(a) > –9
(b) > –6
(c) < 6
(d) < 9
(v) Which of the following is/are an Arithmetic Progression (A.P.)?
1. 1, 4, 9, 16,……….
2. √3, 2√3, 3√3, 4√3,………
3. 8, 6, 4, 2,………
(a) only 1.
(b) only 2.
(c) only 2. and 3.
(d) all 1., 2. and 3.
(vi) The table shows the values of x and y, where x is proportional to y.
| x | 6 | 12 | N |
| y | M | 18 | 6 |
What are the values of M and N?
(a) M = 4, N = 9
(b) M = 9, N = 3
(c) M = 9, N = 4
(d) M = 12, N =0
(vii) In the given diagram,
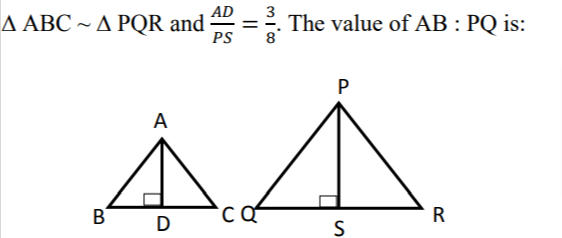
(a) 8 : 3
(b) 3 : 5
(c) 3 : 8
(d) 5 : 8
(viii) A right angle triangle shaped piece of hard board is rotated completely about its
hypotenuse, as shown in the diagram.
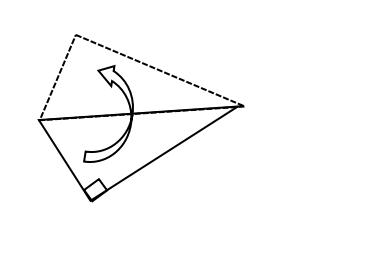
The solid so formed is always:
1. a single cone
2. a double cone
Which of the statement is valid?
(a) only 1.
(b) only 2.
(c) both 1. and 2.
(d) neither 1. nor 2.
(ix) Event A: The sun will rise from east tomorrow.
Event B: It will rain on Monday.
Event C: February month has 29 days in a leap year.
Which of the above event(s) has probability equal to 1?
(a) all events A, B and C
(b) both events A and B
(c) both events B and C
(d) both events A and C
(x) The three vertices of a scalene triangle are always equidistant from a fixed point.
The point is:
(a) Orthocentre of the triangle.
(b) Incentre of the triangle.
(c) Circumcentre of the triangle.
(d) Centroid of the triangle.
(xi) In a circle with radius R, the shortest distance between two parallel tangents is equal
to∶
(a) R
(b) 2R
(c) 2πR
(d) πR
(xii) An observer at point E, which is at a certain distance from the lamp post AB, finds the angle of elevation of top of lamp post from positions C, D and E as α, β and γ. It is given that B, C, D and E are along a straight line.
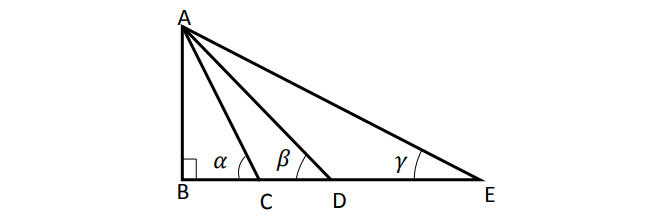
Which of the following condition is satisfied?
(a) tanα > tan β
(b) tan β < tan γ
(c) tan γ > tan α
(d) tan α < tan β
(xiii) 1. Shares of company A, paying 12%, ₹100 shares are at ₹80.
2. Shares of company B, paying 12%, ₹100 shares at ₹100.
3. Shares of company C, paying 12%, ₹100 shares are at ₹120.
Shares of which company are at premium?
(a) Company A
(b) Company B
(c) Company C
(d) Company A and C
(xiv) Which of the following equation represent a line passing through origin?
(a) 3x – 2y + 5 = 0
(b) 2x – 3y = 0
(c) x = 5
(d) y = –6
(xv) For the given 25 variables: 𝒙𝟏 , 𝒙𝟐 , 𝒙𝟑 … … … … … . 𝒙𝟐𝟓
Assertion (A): To find median of the given data, the variate needs to be arranged in ascending or descending order.
Reason (R): The median is the central most term of the arranged data.
(a) A is true, R is false
(b) A is false, R is true
(c) both A and R are true
(d) both A and R are false
Question 2
(i) Shown below is a horizontal water tank composed of a cylinder and two hemispheres. The tank is filled up to a height of 7 m. Find the surface area of the tank in contact with water. Use π = 22/ 7. [4 Marks]
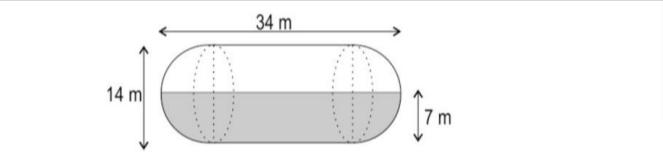
(ii) In a recurring deposit account for 2 years, the total amount deposited by a person is ₹ 9600. If the interest earned by him is one-twelfth of his total deposit, then find: [4 Marks]
(a) the interest he earns.
(b) his monthly deposit.
(c) the rate of interest.
(iii) Find:
(a) (sin 𝜃 + 𝑐𝑜𝑠𝑒𝑐 𝜃)²
(b) (𝑐𝑜𝑠 𝜃 + 𝑠𝑒𝑐 𝜃)²
Using the above results prove the following trigonometry identity.
(sin 𝜃 + 𝑐𝑜𝑠𝑒𝑐 𝜃)² + (cos 𝜃 + sec 𝜃)² = 7 + 𝑡𝑎𝑛2𝜃 + 𝑐𝑜𝑡2𝜃 [4]
Question 3
(i) If a, b and c are in continued proportion, then prove that: [4 Marks]
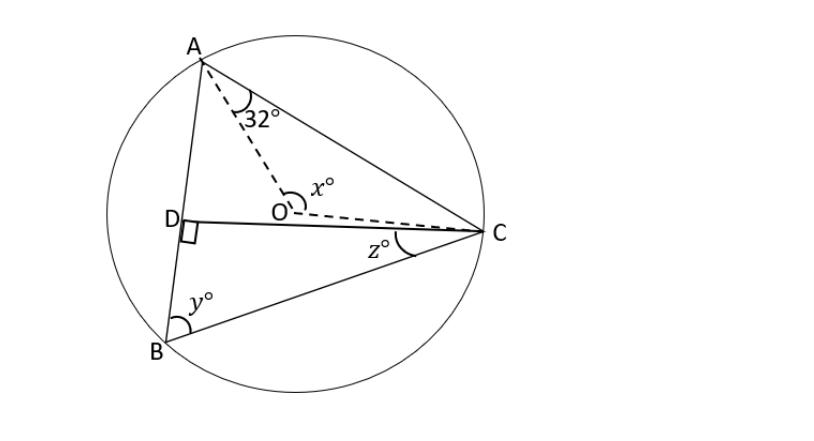
(ii) In the given diagram, O is the centre of circle circumscribing the ∆ABC. CD is perpendicular to chord AB. ∠OAC=32°. Find each of the unknown angles x, y and z.
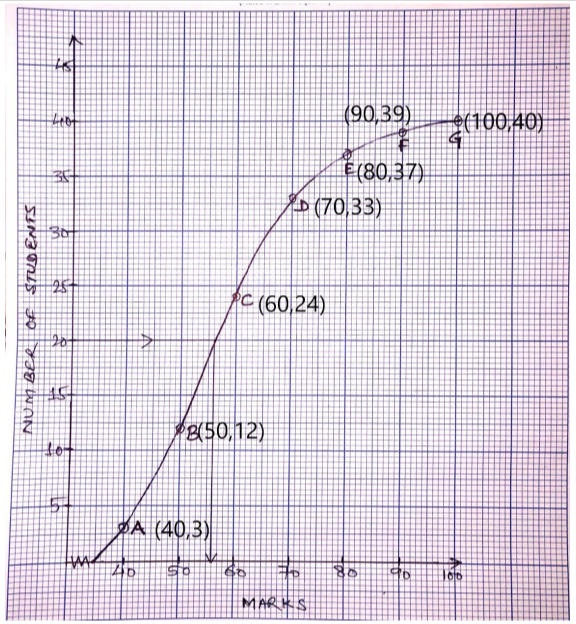
(iii) Study the graph and answer each of the following: [5 Marks]
(a) Name the curve plotted
(b) Total number of students
(c) The median marks
(d) Number of students scoring between 50 and 80 marks
ICSE Class 10 Maths Specimen Paper 2024 Section B
SECTION B
(Attempt any four questions from this Section.)
Question 4
(I) If 𝐴 =
, find 𝐴². If 𝐴² = 𝑝 𝐴, then find the value of 𝑝. [3]
(ii) Solve the given equation 𝑥² − 4𝑥 − 2 = 0 and express your answer correctly to two places
of decimal. (You may use mathematical tables for this question). [3 Marks]
(iii) In the given diagram, ∆ABC is right-angled at B. BDFE is a rectangle. [4 Marks]
AD = 6 cm, CE = 4 cm and BC = 12 cm
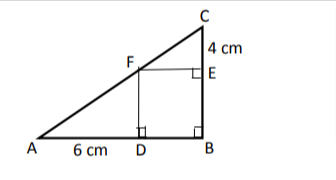
(a) prove that ∆ADF ~∆FEC
(b) prove that ∆ADF ~∆ABC
(c) find the length of FE
(d) find area ∆ADF : area ∆ABC
Question 5
(i) Shown below is a table illustrating the monthly income distribution of a company with 100 employees. [3 Marks]
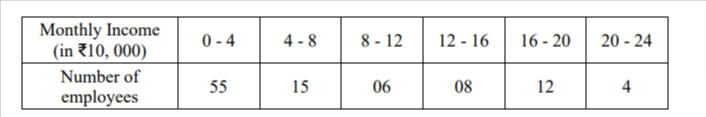
Using step- deviation method, find the mean monthly income of an employee.
(ii) The following bill shows the GST rate and the marked price of articles: [3 Marks]
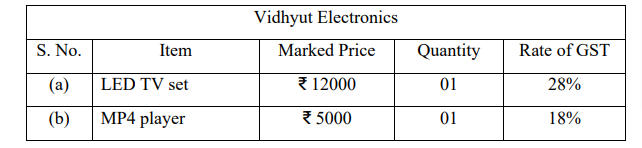
Find the total amount to be paid (including GST) for the above bill.
(iii) In the given figure, O is the center of the circle and AB is tangent to the circle at B.
If ∠PQB= 55º .
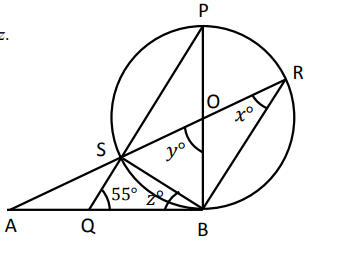
(a) find the value of the angles x, y and z.
(b) prove that RB is parallel to PQ
Question 6
(i) There are three positive numbers in a Geometric Progression (G.P.) such that:
(a) their product is 3375
(b) the result of the product of first and second number added to the product of second and
the third number is 750. Find the numbers. [3]
(ii) The table given below shows the ages of members of a society.
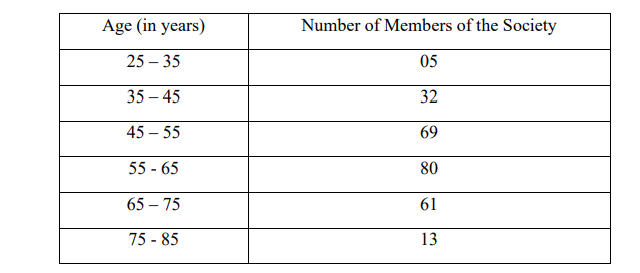
Use graph sheet for this question.
Take 2cm = 10 years along one axis and 2cm=10 members along the other axis.
(a) Draw a histogram representing the above distribution.
(b) Hence find the modal age of the members.
(iii) A tent is in the shape of a cylinder surmounted by a conical top. If the height and radius of
the cylindrical part are 7 m each and the total height of the tent is 14 m. Find the:
(a) quantity of air contained inside the tent.
(b) radius of a sphere whose volume is equal to the quantity of air inside the tent.
Use 𝜋 = 22/ 7 [4]
Question 7
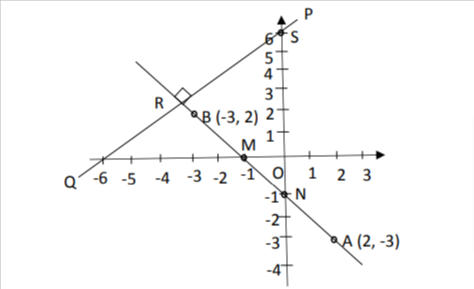
(i) The line segment joining A(2,-3) and B(-3, 2) is intercepted by the 𝑥-axis at the point M
and the y axis at the point N. PQ is perpendicular to AB produced at R and meets the y- axis
at a distance of 6 units from the origin O, as shown in the diagram, at S. Find the:
(a) coordinates of M and N
(b) coordinates of S
(c) slope of AB.
(d) equation of line PQ.
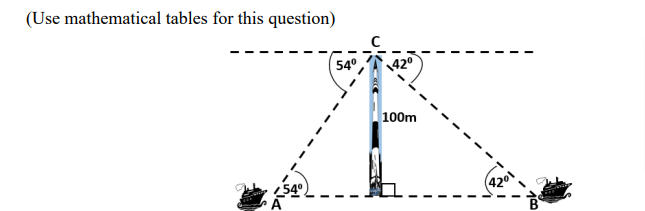
(ii) The angle of depression of two ships A and B on opposite sides of a light house of height
100m are respectively 42º and 54º . The line joining the two ships passes through the foot
of the lighthouse.
(a) Find the distance between the two ships A and B.
(b) Give your final answer correct to the nearest whole number.
Question 8
(i) Solve the following inequation write the solution set and represent it on the real number line. [3]
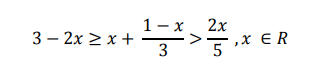 (ii) ABCD is a cyclic quadrilateral in which BC = CD and EF is a tangent at A.
(ii) ABCD is a cyclic quadrilateral in which BC = CD and EF is a tangent at A.

∠CBD = 43° and ∠ADB = 62°. Find:
(a) ∠ADC
(b) ∠ABD
(c) ∠FAD
(iii) A (a, b), B(-4, 3) and C(8,-6)are the vertices of a ∆ABC. Point D is on BC such that
BD : DC is 2 : 1 and M (6, 0) is mid point of AD. Find:
(a) coordinates of point D.
(b) coordinates of point A.
(c) equation of a line passing through M and parallel to line BC. [4]
Question 9
(i) Using componendo and dividend, find the value of x, when:
[3]
(ii) The total expense of a trip for certain number of people is ₹18000. If three more people join
them, then the share of each reduces by ₹3000. Taking x to be the original number of people,
form a quadratic equation in x and solve it to find the value of x.
(iii) Using ruler and compass only construct ∠ABC = 60°, AB = 6 cm and BC = 5 cm.
(a) construct the locus of points equidistant from AB and BC.
(b) construct the locus of points equidistant from A and B.
(c) Mark the point which satisfies both the conditions (a) and (b) as P.
Hence, construct a circle with centre P and passing through A and B. [4]
Question 10
(i) Using remainder and factor theorem, factorize completely, the given polynomial:
2𝑥³ − 9𝑥² + 7𝑥 + 6 [3]
(ii) Each of the letter of the word “HOUSEWARMING”” is written on cards and put in a bag.
If a card is drawn at random from the bag after shuffling, what is the probability that the letter on the card is∶
(a) a vowel
(b) one of the letters of the word SEWING.
(c) not a letter from the word WEAR. [3]
(iii) Use a graph sheet for this question. Take 2 cm = 1 unit along the axes.
(a) Plot A (1, 2), B(1, 1), and C (2, 1)
(b) Reflect A, B, and C about the y-axis and name them as Aʹ, Bʹ, and Cʹ.
(c) Reflect A, B, C, Aʹ, Bʹ, and Cʹ about the x-axis and name them as Aʺ, Bʺ, Cʺ, Aʺʹ, Bʺʹ
and Cʺʹ respectively.
(d) Join A, B, C, Cʺ, Bʺ, Aʺ, Aʺʹ, Bʺʹ, Cʺʹ, Cʹ, Bʹ, Aʹ and A to form a closed figure.



 ICSE English Language Specimen Paper 202...
ICSE English Language Specimen Paper 202...
 ICSE Class 10 Commercial Studies Specime...
ICSE Class 10 Commercial Studies Specime...
 ICSE Class 10 Environmental Science Spec...
ICSE Class 10 Environmental Science Spec...





























