Integration of Tan x – Explanation, Formula, Proof, Examples

Integration of Tan x: The integration of trigonometric ratios is one of the crucial topics in the field of trigonometry domain of mathematics. One such integration is the integration of Tan x. Tan x is one of the six trigonometric ratios apart from Sin x, Cos x, Cosec x, Cot x, and Sec x. Tan x is equal to the ratio of the perpendicular and base. In a right-angled triangle, the side opposite to the angle “x” will be called perpendicular while the other side (other than hypotenuse) will be known as the base. Let us understand this concept in a more detailed way by exploring various aspects of its integration.

Integration of Tan x

The integration of Tan x means summing the values of this ratio in the defined range. It is also known as the integral of trigonometric function Tan x. It is denoted by ∫(Tan x) dx, which means the integration/integral of Tan x with respected to x. The integration of Tan x gives us Sec x in its result. The formula for Tan x is given by:

Tan x = perpendicular/base = Sin x/Cos x

where, x is the angle in consideration

Before exploring its integral formula and the proof for the same, let us first understand what exactly is integration.

What is Integration?

Integration is the opposite function of differentiation. A function’s integral can be found through the process of integration. The accumulation or addition of values over an interval is represented by the integral of a function. Finding the area beneath a curve is the essence of integration, to put it simply. It enables us to compute the overall impact or accumulative quantity of a changing quantity, which is frequently depicted as a function. It is denoted by the symbol ∫. As we have understood the basic of integration, let us get acquainted with the formula of the integration of Tan x.

Integration of Tan x Formula

As discussed above, the integration is the opposite function of the differentiation. So, the result of integration of a function is not equal to the result of the derivative of the function. It must be noted that the function Tan x is continuous for all real numbers except values = (2n+ 1). Π/2. Also, the domain of Tan x = Range of Tan x, except for the values (2n+ 1). Π/2. The formula for the integral of Tan x is shown below.

The integration of Tan x is given by:

∫(Tan x) dx = ln |Sec x| + C

where, ∫(Tan x) dx = integration of Tan x with respect to x

ln = natural logarithm

C = any constant

As you can see from the above formula that the integral of Tan x results in Sec x. we can also represent the result of the integral of Tan x in the form of Cos x. The same has been done below.

Integration of Tan x in terms of Cos x

The integral of Tan x can be represented in terms of Cos x too. As we know that Sec x is the inverse trigonometry ratio of Cos x. That is, Sec x = 1/Cos x. So, we can find the integral result of Tan x in terms of Cos x.

The integration or Tan x is terms of Cos x is expressed as:

∫(Tan x) dx = -ln |Cos x| + C

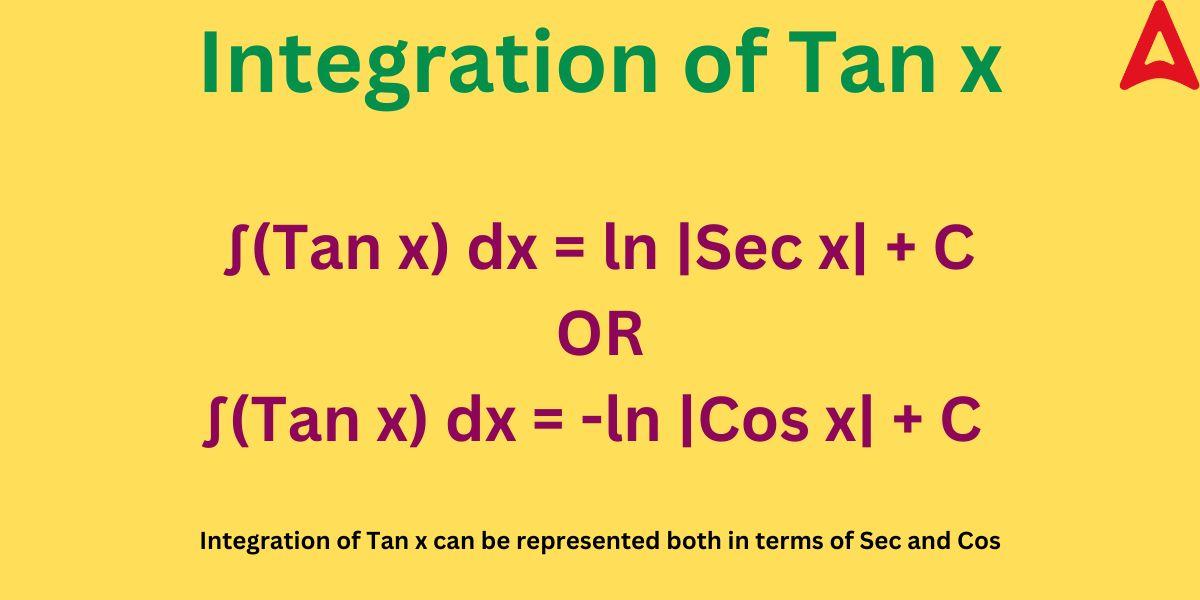
Hence, we can see that the integral value of Tan x can be represented both in terms of Sec x and Cos x. The only difference between these results is the negative sign. This is so because Sec x is the inverse ratio of Cos x.

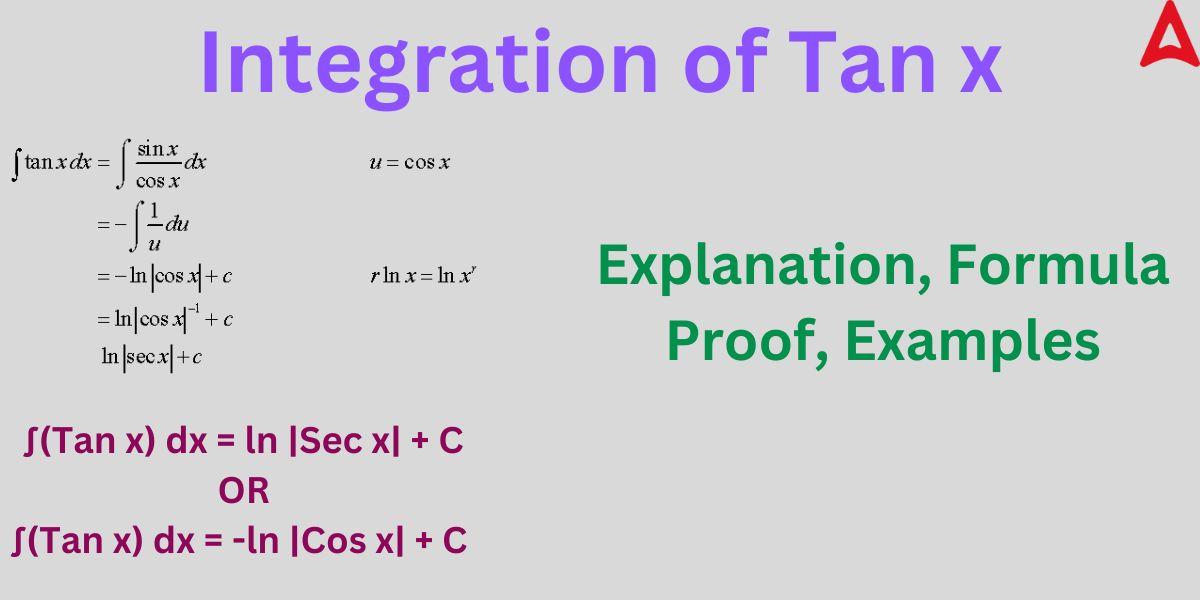
Integration of Tan x Proof

We can find the result received after the integration of Tan x by the following method

Here, f(x) = Tan x

∫f(x) dx = ∫(Tan x) dx

as Tan x = Sin x/Cos x

so, ∫(Tan x) dx = ∫ (Sin x/Cos x) dx

or, (Tan x) dx = ∫ (1/Cos x). Sin x dx ———-(i)

We will now apply the substitution rule of integration.

Let b = Cos x

So, db = – Sin x dx

Sin x dx = -db

putting this value of Sin x dx in equation (i), we get

∫ Tan x dx = ∫(Sin x /Cos x) dx = ∫ (1/b) (-db) = – ∫ (1/b) db

as the integration of 1/b = ln b + C, where ln = natural log and C = constant

so, ∫ Tan x dx = – ln |b| + C

= -ln |Cos x| + C

as  x log(y) = log(y)^x

= ln |(Cos x)^-1| + C

= ln |1/Cos x| + C

= ln |Sec x| + C

Therefore, ∫ Tan x dx = -ln |Cos x| + C = ln| Sec x| + C

Definite Integration of Tan x

The definite integration or definite integral is the integration of a function in a given range. That is, a definite integral integrates the given function form one point to another. The starting point or value is known as the lower limit while the end point or value is known as the upper limit. The definite integral is denoted as

f(x) dx. Here, a is the lower limit while b is the upper limit. The expansion of the definite integral will give the result as follows:

f(x) dx = f(q) -f(p)

so,

Tan x dx = [ ln | Sec q| – ln | Sec p|] + C

Integration of Tan x Graph

we can represent the integral values of Tan x in a graphical form. The same has been depicted below.

As we can clearly observe from the graph that the graph is non-continuous at odd multiples of Π/2. It is because, the Tan x is undefined at these values.

Integration of Tan x Class 12

The integral of Tan x is an important topic in class 12 Mathematics syllabus of NCERT. It is important to remember the formula for the integration of Tan x for class 12. Apart from that, students should also go through the solved examples given in the next section to have a thorough understanding of this topic. Some important results from the integral of Tan x for class 12 students is listed below.

∫(Tan x) dx = ln |Sec x| + C

or, ∫(Tan x) dx = – ln |Cos x| + C

for convenience and calculation purposes, we can replace the ln with log

Hence, ∫(Tan x) dx = log |Sec x| + C = – log |Cos x| + C

Integration of Tan x Examples

Some of the solved examples related to the integration of Tan x is given below for students. These solved questions will help students understand this concept in a better way.

Example 1: Find the value of

.

Solution: As we know that the integration of Tan x dx gives – log | Cos x| + C

so by using the definite integral rule,

= – [log | Cos 30| – log | Cos 0|] + C

as Cos 30 = √3/2 and Cos 0 = 1

so, – log (√3/2) + log (1)

using the division rule of log and log 1 =0, we get

– log (√3) + log(2) + 0

or, log 2 – (1/2) log 3

Hence, the result of the question = log 2 – (1/2) log 3

Example 2: Find the integral value of Tan²x

solution: given f(x) = Tan²x

∫f(x) dx = ∫Tan²x dx

as Sec²x – 1 = Tan²x

so, ∫f(x) dx = ∫(Sec²x – 1) dx

or, ∫Sec²x dx – ∫1 dx

as Sec²x dx = Tan x and 1 dx = x

= Tan x – x + C

Hence, ∫Tan²x dx = Tan x – x + C

Example 3: Find the integration of tan x . sec x

Solution: given f(x) = tan x . sec x

∫f(x) dx = ∫ (tan x. sec x) dx

as tan x = cos x/sin x  and sec x = 1/cos x

so, ∫(tan x . sec x) dx = ∫(sin x/cos x)(1/cos x) dx

or, ∫(sin x/cos²x) dx

Let cos x = t.

Taking derivatives on both sides, we get

-sin x dx = dt (because the derivative of cos x = – sin x)

or, sin x dx = – dt

putting these values in place of ∫(sin x/cos²x) dx, we have

∫(tan x . sec x) dx = ∫(-1/t²) dt

As ∫xⁿ = xⁿ⁺¹/(n + 1)+ C

= (-t^-1/(-1)) + C

= (1/t) + C

putting cos x in place of t

= 1/cos x + C

= sec x + C

Hence, ∫ (tan x. sec x) dx = sec x + C

Sharing is caring!