Lens Formula- Magnification, Power of Lens Formula Derivation

The lens formula is applicable for both types of lenses, concave and convex lenses. lens Formula is 1/u + 1/v = 1/f Derivation of this is available here.

Table of Contents

Lens

A lens is an optical device that is designed to focus or redirect light rays. Lenses are commonly used in various optical instruments and devices, including cameras, eyeglasses, microscopes, telescopes, and binoculars. They are made from transparent materials, such as glass or plastic, and have curved surfaces that cause light to either converge (converging lens) or diverge (diverging lens).

There are two main types of lenses:

Convex Lens (Converging Lens): This type of lens is thicker at the center than at the edges. Convex lenses are designed to bring parallel rays of light together at a single point called the focal point. They are commonly used in devices like magnifying glasses, cameras, and telescopes to create real and inverted images.

Concave Lens (Diverging Lens): Concave lenses are thinner at the center and thicker at the edges. They cause parallel rays of light to spread out as if they were coming from a single point behind the lens. Concave lenses are often used in correcting vision problems in eyeglasses and contact lenses.

Lenses are fundamental in the field of optics and play a crucial role in manipulating and focusing light for various applications in science, technology, and everyday life. They can be combined in optical systems to create more complex optical elements, such as compound lenses, which have multiple lens elements designed to achieve specific optical effects.

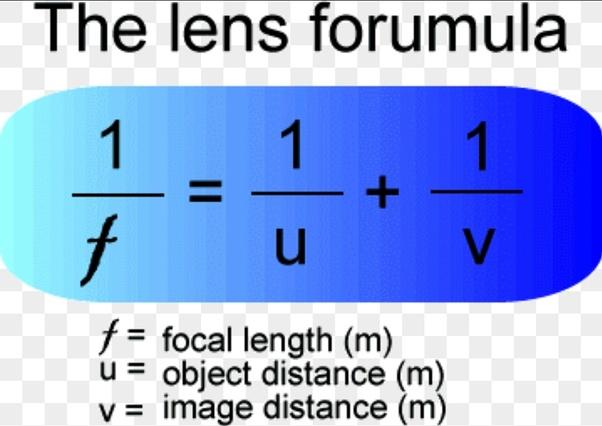
Lens Formula

The lens formula is given below. The Lens formula can be used to calculate the distance between the image and the lens.

1/u + 1/v = 1/f.

V = distance of the image from the lens.

U=distance of an object from the lens.

F=focal length.

The lens formula applies to all situations. If the equation results in negative image distance, then the image is virtual. If the equation shows a negative focal length then the lens is a diverging lens.

What is a Lens formula?

Lens formula- A lens is a piece of glass that either concentrate the rays of light at a point or it diverges the light from one concentrated point. It does the converging or diverging with the help of dispersion. A simple lens consists of a transparent material while the compound lens consists of several lenses.

Lenses can be broadly classified into two types. They are convex lens and concave lens. A convex lens is also known as a converging lens. The reason is when rays of light fall on the lens, the rays get converged when refracted back.

A concave lens is called a diverging lens as light rays diverge apart after falling on the lens. Images formed by the convex lens can either be real or virtual. It depends upon factors like the distance of the image from the lens and the size of the lens and the object.

The distance between the image and the lens can be calculated by the focal length and the object distance using the lens formula.

Lens Formula Derivation: Lens Maker Formula

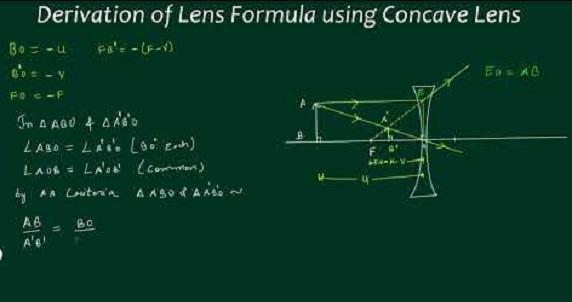
derivation of marker lens formula — Derivation of Marker Formula

Below we have pointed the derivation of the lens formula.

First surface

n2/v1 –n2/v2=n2-n1/r…………………………1

Second surface

N1/v –n2/v1=n1-n2/r2………………………2

Adding 1 and 2 we get,

1/v-1/u= (n2/n1-1)[1/R1-1/R2]

Also,

1/v-1/u=1/f

Hence, 1/f= (refractive index-1)(1/R1-1/R2)

Check: CSA of a cylinder formula

Power of Lens Formula

The power of a lens is a measure of its ability to converge or diverge light. It is typically expressed in units called diopters (D) and is calculated using the following formula:

$P =1/ f$

Where:

$P$ is the power of the lens in diopters (D).
$f$ is the focal length of the lens in meters (m).

In this formula, a positive power ( $P$ ) indicates that the lens is a converging lens (convex lens), which brings parallel rays of light together at a focal point. A negative power ( $P$ ) indicates that the lens is a diverging lens (concave lens), which causes parallel rays of light to diverge as if they came from a focal point.

The greater the absolute value of the power of a lens, the stronger its ability to converge or diverge light. For example, a lens with a power of +2D is stronger (more converging) than a lens with a power of +1D, while a lens with a power of -2D is stronger (more diverging) than a lens with a power of -1D.

You might be interested in: Cos 60 degree calculation

Magnification Formula for Lens

The magnification formula for a lens is given by:

$M = - u v$

Where:

$M$ is the magnification of the lens.
$v$ is the image distance, which is the distance between the image and the lens. If the image is real and formed on the same side as the object, $v$ is considered negative. If the image is virtual and formed on the opposite side of the object, $v$ is considered positive.
$u$ is the object distance, which is the distance between the object and the lens.

Lens Formula Examples

lens formula some examples are given below.

If an object is 12cm from a concave lens with a focal length of 6cm what is the image distance from the lens and is the image upright or upside down?

Solution:

=> F=do+di

=> 1-6=112+di

=> -0.166=0.0833+di

=> di=-0.25

=> 1 -0.25=di

=> di=-4

All concave images are upright.

Hence solution is −4 and Upright.

FAQs