Logarithm is an alternate way of writing the exponential function. It is defined as the power to which a base number of the logarithm must be raised to get the given number.

What will be the value of log(0).

The value of log(0) is undefined.

Why do we use logarithm formula?

Logarithms can be used to solve complex problems that are not easy to solve with the exponential function. Wo we use the logarithm formula to derive the log values for solving complex problems.

Logarithm Formula- Explanation, Types, Properties, Examples -

Table of Contents

The logarithm Formula is one of the most widely used formulas used for solving complex calculations. Logarithm, also known commonly by the name log, is basically an alternative way to write the exponents. Mathematical calculations and many other scientific disciplines depend heavily on logarithms. A mathematical idea known as the logarithm is utilized frequently in fields including physics, engineering, computer science, and finance to assist in simplifying complicated computations. This formula is used to find the values related to logs.

Logarithm Formula

The logarithm formula is an important formula for solving complex problems in higher classes related to exponents. This formula is used whenever the concept of logarithm comes into play. Before understanding this formula, let us first have a deeper look about logarithms.

What are Logarithms?

Logarithms are another method in which an exponential number can be expressed. It is the opposite function of the exponential function just like the division is opposite that of the multiplication. Simply put, it is the power to which a base number of the logarithm must be raised to get the given number. You will have a clear understanding of this concept by going through the explanation given below.

The logarithm formula for an exponential expression a^b= c is given by log_a(c) = b. Some of the examples of exponent form to log form is given below.

3⁶= 729 can be written in logarithm form as log₃(729) = 6

4⁴= 256 can be written in logarithm form as log₄(256) = 4

p^q= a can be written in logarithm form as log_p(a) = q

2¹⁰= 1024 can be written in logarithm form as log₂(1024) = 10

Logarithm Formula Explanation

This formula is used to derive the values of exponential functions by converting them into the logarithmic functions. It makes calculation quite easy, hence saving a lot of effort. Let us understand this formula in detail.

The logarithmic form of an exponent is written in the following way.

Let us suppose we have been given x^y= z

then, the logarithm of the above exponential function becomes

log_x(z) = y

where, x is known as the base of the log

z is known as the argument of the log

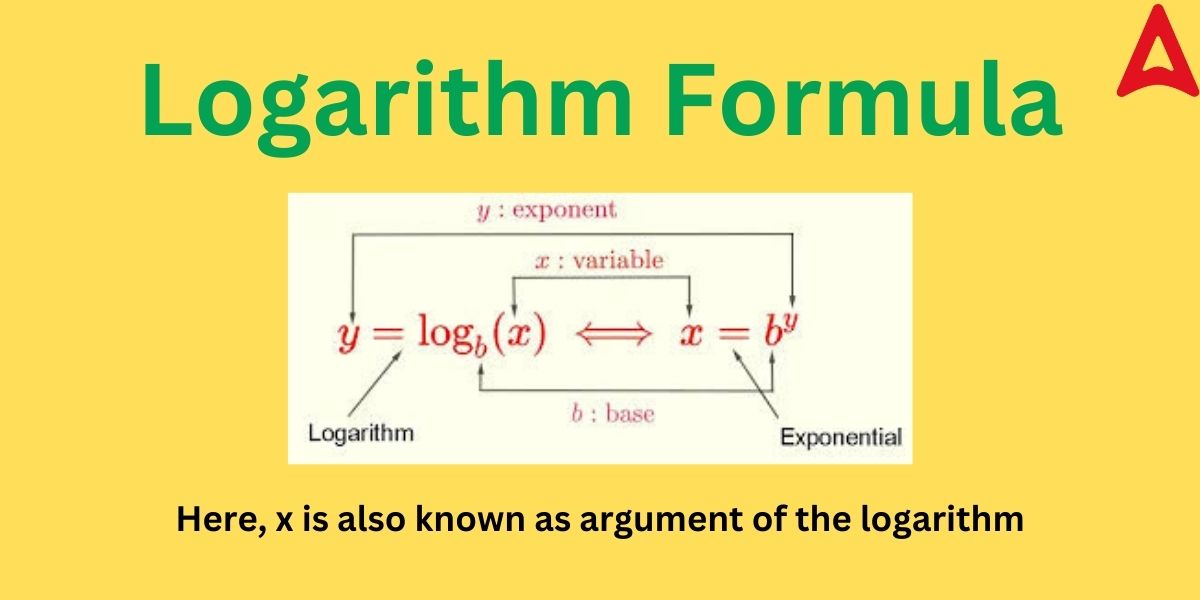
As you can observe from the above formula and diagram, the base of the log is the number to which the exponent is raised.

Logarithm Formula in Hindi

Logarithm को हिंदी में लघुगणक कहते हैं| लघुगणक सूत्र का उपयोग घातीय कार्यों को लघुगणकीय कार्यों में परिवर्तित करके उनके मान प्राप्त करने के लिए किया जाता है। यह गणना को काफी आसान बना देता है, जिससे बहुत सारा प्रयास बच जाता है। आइए इस फॉर्मूले को विस्तार से समझते हैं.

किसी घातांक का लघुगणकीय रूप निम्नलिखित प्रकार से लिखा जाता है।

मान लीजिए कि हमें a^b= c दिया गया है

फिर, उपरोक्त घातीय फलन का लघुगणक बन जाता है

log_a(c) = b

जहां, a को लघुगणक के आधार के रूप में जाना जाता है

c को लघुगणक के तर्क के रूप में जाना जाता है

जिसे अंग्रेजी भाषा में Argument कहते हैं, उसे हिन्दी भाषा में तर्क कहा जाता है जबकी base को हिन्दी भाषा में आधार कहा जाता है|

जैसा कि आप उपरोक्त सूत्र से देख सकते हैं, लघुगणक का आधार वह संख्या है जिस पर घातांक बढ़ा हुआ है।

Logarithm Formula Types

There are basically two types of logarithms. The value for both these types of logarithms can be found using this formula. The two types of logarithm are stated below.

1) Natural Logarithm: Natural logarithm are those logarithm that always contain a constant “e” in its base. This constant is known as Euler’s constant. It is therefore also recognized as base “e” constant. It is denoted by loge or ln. The value of “e” is about 2.718. In other words, by what power the “e” must be raised to get the desired number is defined by the natural logarithm.

Example: ln(2) = 0.693

ln(5) = 1.61

2) Common Logarithm: The common logarithm are those logarithm that has base as 10. It is denoted as log₁₀or simply log. It is therefore also recognized as base 10 logarithms. In simple words, by what power 10 must be raised so as to get the desired number is defined by the common logarithm.

Example: log (100) = 2

log₁₀(1000) = 3

Common and Natural Logarithm Formula Relation

The common logarithm and the natural logarithm are connected to each other.

The relation between them is given by the formula:

ln = 2.303 x log

where, ln = natural logarithm

log = common logarithm with base 10

Logarithm Formula Properties

There exist some unique properties in the logarithm formula that is useful in understanding various logarithmic problems. Some of the unique properties of the logarithm formula is given below:

log_a(a) = 1, i.e., if the base and argument is same then the answer is always 1

log 1 = 0

log 0 = undefined

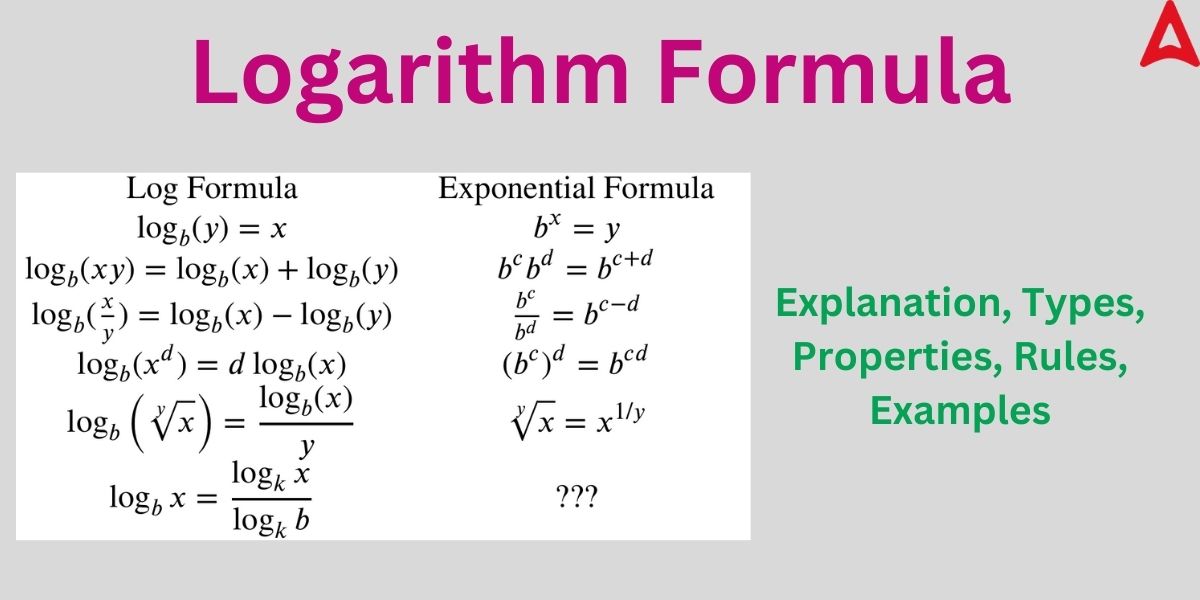
Basic Logarithm Formula

Some of the logarithm formula are used ubiquitously to solve various problems. These are also known as basic formulas in the logarithmic world. These basic formulas are listed below.

log(ab) = log(a) + log(b)
log(a/b) = log(a) – log(b)
log(a)^b= b log(a)
log(√a) = log (a)^1/2= (1/2) log(a)
log(a^x/b^y) = x log(a) – y log(b)
log(a^xb^y) = x log(a) + y log(b)

Logarithm Formula Class 11 and Class 12

The logarithm formula is extensively used in various calculations in class 11 and class 12. Students of these classes must be familiar with these formulas so as to solve various complex problems. Some formulas related to logarithm essential for class 11 and class 12 students are given below:

log 1 = 0

log(ab) = log(a) + log(b)

log(a/b) = log(a) – log(b)

log 0 = undefined

log(a + b) = log a + log(1+ba)

log(a – b) = log a + log (1-b/a)

log_a(a) = 1

log(a)^b= b log(a)

log(

\sqrt[m]{b}

) = (1/m) log(b)

Logarithm Formula Rules

The logarithm formula is governed by some rules. These rules are used to deduce the values of logs in different cases. Some of these rules are stated herein:

1) Base Switch Rule

Using this rule, we can switch the base of the log with the argument.

log_a(b) = 1/log_b(a)

2) Log Integral Rule

This rule states the value of log in case of integration.

∫log_a(y)dy = y( log_a(y) – 1/ln(a) ) + C

3) Log Derivative Rule

This rule helps us to find the derivative of a logarithm function

If f(y) = log_a(y)

Then, the derivative of f(y), i.e., f'(y) will be

f'(y) = 1/(y (ln(a)))

4) Log Product Rule

This rule deals with the cases when two numbers are in multiplication in the logarithm

log(ab) = log(a) + log(b)

5) Log Division Rule

As per log division rule:

log (a/b) = log(a) – log(b)

6) Log Identity Rule

It states that if the base and argument of the log is same then the answer is always 1

log_a(a) = 1

7) Log exponential Rule

log(a)^b= b log(a)

8) Logarithm in Power Rule

According to this rule, if a logarithm with base “a” is raised to the power “a”, then the answer will be equal to the argument of the log function.

Mathematically, a^log(x) = x

where log(x) has the base “a”

9) Log Zero Value Rule

The value of Log 1 is always 0 no matter what the base is.

log (1) = 0

10) Log Undefined Rule

log (0) = undefined

Logarithm Formula for JEE

The competitive examination of JEE (Joint Entrance Examination) involves several complex calculations. The logarithm formula comes as a handy tool for such calculations. So, it is advisable for students of JEE to prepare and memorize the logarithm formulas given below to ace their examinations.