Math Symbols: We are unable to visualize mathematics without math symbols, whether it’s a simple addition problem or a difficult calculus problem. These mathematical symbols are necessary for various mathematical processes. These math symbols are employed in a variety of mathematical domains. from the representation of the equation to the explanation of what’s going on between the two integers. In mathematical operations, all math symbols are utilized to represent diverse concepts. Mathematical dimensions include algebra, trigonometry, geometry, and number theory, and the concept of maths is entirely based on numbers and math symbols. So in this article we dive into different sorts of math symbols of various math chapters such as Algebra, Sets, probability, calculus, math symbols greek, numerical symbols and more.
Math Symbols
It’s worth noting that Mathematics is entirely dependent on numbers and math symbols. In various conceptions, the primary use of all math symbols is to carry out mathematical operations.There are numerous other uses for math symbols besides referring to distinct quantities.
- Math symbols in many topics and It aids in the representation of quantities.
- Establishes quantitative relationships.
- It aids in determining the type of operation we must carry out.
Mathematical Symbols with Names
Math symbols are ubiquitous and help to bridge the language gap. It all revolves around numbers, symbols, and formulas in mathematics. To express mathematical ideas, basic mathematical symbols are utilised. The symbol-value relationship relates to the fundamental mathematical condition. We’re here talking about the fundamental mathematical symbols with Names that we are learning from our childhood.
| Basic Math symbols | |||
| Math Symbol | Symbol Name in Maths | Meaning | Example |
| ≠ | not equal sign | inequality | 9 ≠ 5 |
| = | equal sign | equality | 10 = 8 + 2 |
| < | strict inequality | less than | 9 < 18 |
| > | strict inequality | greater than | 8 > 1 |
| ≤ | inequality | less than or equal to |
x ≤ y, means, y = x or y > x
|
| ≥ | inequality | greater than or equal to |
a ≥ b, means, a = b or a > b, but not vice-versa
|
| − | minus sign | subtraction | 8 − 2 = 6 |
| + | plus sign | addition | 9 + 2 = 11 |
| ∓ | minus – plus | both minus and plus operations |
1 ∓ 4 = -3 and 5
|
| ± | plus – minus | both plus and minus operations |
5 ± 3 = 8 and 2
|
| × | times sign | multiplication | 4 × 3 = 12 |
| [ ] | brackets | calculate expression inside first |
[ 3×6] + 2 = 20 + 3 = 23
|
| ( ) | parentheses | calculate expression inside first |
3 × (3 + 7) = 3 × 10 = 30
|
| n√a | n-th root (radical) | n√a · n√a · · · n times = a |
for n=3, n√8 = 2
|
| ppm | per-million | 1 ppm = 1/1000000 |
10ppm × 30 = 0.0003
|
| ‰ | per-mille | 1‰ = 1/1000 = 0.1% |
10‰ × 30 = 0.3
|
| ppt | per-trillion | 1ppt = 10-12 |
10ppt × 30 = 3×10-10
|
| ppb | per-billion | 1 ppb = 1/1000000000 |
10 ppb × 30 = 3×10-7
|
| * | asterisk | multiplication | 2 * 3 = 6 |
| ÷ | division sign / obelus | division | 15 ÷ 5 = 3 |
| ∙ | multiplication dot | multiplication | 2 ∙ 3 = 6 |
| – | horizontal line | division / fraction | 8/2 = 4 |
| / | division slash | division | 6 ⁄ 2 = 3 |
| mod | modulo | remainder calculation | 7 mod 3 = 1 |
| ab | power | exponent | 24 = 16 |
| . | period | decimal point, decimal separator |
4.36 = 4 +(36/100)
|
| √a | square root | √a · √a = a | √9 = ±3 |
| a^b | caret | exponent | 2 ^ 3 = 8 |
| 4√a | fourth root | 4√a ·4√a · 4√a · 4√a = a | 4√16= ± 2 |
| 3√a | cube root | 3√a ·3√a · 3√a = a | 3√343 = 7 |
| % | percent | 1% = 1/100 | 10% × 30 = 3 |
Math Symbols List Logical
To execute various processes, a logical math symbols list is employed. The logical math symbols make referring to mathematical quantities easier. The logical math symbols not only relate to distinct quantities, but they also express the connections between two quantities and help to concise the interpretation.
| Logical Math Symbols | |||
| Math Symbols | Symbol Name in Math | Meaning | Example |
| ⋅ | and | and | x ⋅ y |
| & | ampersand | and | x & y |
| + | plus | or | x + y |
| ^ | caret / circumflex | and | x ^ y |
| ⇔ | equivalent |
if and only if (iff)
|
– |
| ↔ | equivalent |
if and only if (iff)
|
– |
| ∨ | reversed caret | or | x ∨ y |
| | | vertical line | or | x | y |
| x’ | single quote | not – negation | x’ |
| x | bar | not – negation | x |
| ¬ | not | not – negation | ¬ x |
| ! | exclamation mark | not – negation | ! x |
| ⊕ | circled plus / oplus | exclusive or – xor | x ⊕ y |
| ~ | tilde | negation | ~ x |
| ⇒ | implies | – | – |
| ∴ | therefore | – | – |
| ∵ |
because / since
|
– | – |
| ∀ | for all | – | – |
| ∃ | there exists | – | – |
| ∄ |
there does not exists
|
– | – |
Math Symbols Greek
We frequently employ Greek symbols in other subjects as well. In their work, mathematicians utilize Greek alphabets and math symbols Greek to denote variables, constants, functions, and so on. The names of some of the most regularly used Greek symbols in mathematics are mentioned below.
| Math Symbols Greek | ||||
| Upper Case Letter | Lower Case Letter | Greek Letter Name | How to Pronounce |
English Equivalent/ Meaning
|
| Α | α | Alpha | al-fa | a |
| Β | β | Beta | be-ta | b |
| Γ | γ | Gamma | ga-ma | g |
| Δ | δ | Delta | del-ta | d |
| Ε | ε | Epsilon | ep-si-lon | e |
| Ζ | ζ | Zeta | Ze-ta | z |
| Η | η | Eta | eh-ta | h |
| Θ | θ | Theta | te-ta | th |
| Ι | ι | Iota | io-ta | i |
| Κ | κ | Kappa | ka-pa | k |
| Λ | λ | Lambda | lam-da | l |
| Μ | μ | Mu | m-yoo | m |
| Μ | μ | Mu | m-yoo | m |
| Ν | ν | Nu | noo | n |
| Ν | ν | Nu | noo | n |
| Ξ | ξ | Xi | x-ee | x |
| Ο | ο | Omicron | o-mee-c-ron | o |
| Π | π | Pi | pa-yee | p |
| Ρ | ρ | Rho | row | r |
| Σ | σ | Sigma | sig-ma | s |
| Τ | τ | Tau | ta-oo | t |
| Υ | υ | Upsilon | oo-psi-lon | u |
| Φ | φ | Phi | f-ee | ph |
| Χ | χ | Chi | kh-ee | ch |
| Ψ | ψ | Psi | p-see | ps |
| Ω | ω | Omega | o-me-ga | o |
Geometry Symbols
Geometry is an important subject of mathematics that is concerned with the features of geometric object configurations such as lines that are parallel, angles, circles, points, and so on. Here we include all of the geometry symbols that students should be familiar with.
| Geometry Symbols in Mathematics | |||
| Math Symbol | Symbol Name | Meaning | Example |
| deg | degree | 1 turn = 360deg | α = 60deg |
| ∠ | angle | formed by two rays | ∠ABC = 30° |
| ° | degree | 1 turn = 360° | α = 60° |
|
measured angle
|
the angle we are talking about | ABC = 30° | |
|
spherical angle
|
AOB = 30° | ||
| ∟ | right angle | α = 90° | |
| grad | gradians/gons | grads angle unit | 360° = 400 grad |
| g | gradians/gons | grads angle unit | 360° = 400 g |
| ′ | prime | arcminute, 1° = 60′ | α = 60°59′ |
| ″ | double prime | arcsecond, 1′ = 60″ | α = 60°59′59″ |
| line | infinite line | ||
| AB | line segment |
line from point A to point B
|
|
| ray |
line that start from point A
|
||
| arc |
arc from point A to point B
|
||
| ⊥ | perpendicular | perpendicular lines (90° angle) | AC ⊥ BC |
| ∥ | parallel | parallel lines | AB ∥ CD |
| ≅ | congruent to | equivalence of geometric shapes and size | ∆ABC≅ ∆XYZ |
| ~ | similarity | same shapes, not same size | ∆ABC~ ∆XYZ |
| Δ | triangle | triangle shape | ΔABC≅ ΔBCD |
| |x-y| | distance | distance between points x and y | | x-y | = 5 |
| π | pi constant | π = 3.141592654…is the ratio between the circumference and diameter of a circle | c = π⋅d = 2⋅π⋅r |
| rad | radians | radians angle unit | 360° = 2π rad |
| c | radians | radians angle unit | 360° = 2π c |
Algebra Symbols
These math symbols in algebra indicate non-fixed quantities known as variables. Algebra is a mathematical aspect that involves symbols and the rules used to deceive those symbols. Mathematics discusses the link between variables in algebra. Look at the table below, where we discussed almost all algebra symbols.
| Algebra Symbols | |||
| Math Symbol | Symbol Name | Meaning | Example |
| f (x) | function of x | maps values of x to f(x) | f (x) = 3x+5 |
| | x | | single vertical bar | absolute value | | -5 | = 5 |
| ≡ | equivalence | identical to | |
| x | x variable | unknown value to find |
when 2x = 4, then x = 2
|
| := | equal by definition |
equal by definition
|
|
| ≜ | equal by definition |
equal by definition
|
|
| ≈ | approximately equal | approximation |
sin(0.01) ≈ 0.01
|
| ~ | approximately equal | weak approximation | 11 ~ 10 |
| ∞ | lemniscate |
infinity symbol
|
|
| ∝ | proportional to | proportional to |
y ∝ x when y = kx, k constant
|
| ≫ | much greater than | much greater than | 1000000 ≫ 1 |
| ≪ | much less than | much less than | 1 ≪ 1000000 |
| [ ] | brackets | calculate expression inside first |
[(1+2)*(1+5)] = 18
|
| ( ) | parentheses | calculate expression inside first | 2 * (3+5) = 16 |
| ⌊x⌋ | floor brackets | rounds number to lower integer | ⌊4.3⌋= 4 |
| { } | braces | set | |
| x! | exclamation mark | factorial |
4! = 1*2*3*4 = 24
|
| ⌈x⌉ | ceiling brackets | rounds number to upper integer | ⌈4.3⌉= 5 |
| π | pi constant | π = 3.141592654… is the ratio between the circumference and diameter of a circle |
c = π·d = 2·π·r
|
| φ | golden ratio |
golden ratio constant
|
|
| (a,b) | open interval | (a,b) = {x | a < x < b} | x ∈ (2,6) |
| (f ∘g) | function composition | (f ∘g) (x) = f (g(x)) |
f (x)=3x, g(x)=x-1 ⇒(f ∘g)(x)=3(x-1)
|
| ∆ | delta | change / difference | ∆t = t1 – t0 |
| [a,b] | closed interval | [a,b] = {x | a ≤ x ≤ b} | x ∈ [2,6] |
| ∑ | sigma | summation – sum of all values in range of series |
∑ xi= x1+x2+…+xn
|
| ∆ | discriminant | Δ = b2 – 4ac | |
| ∑∑ | sigma |
double summation
|
|
| e | e constant / Euler’s number | e = 2.718281828… |
e = lim (1+1/x)x , x→∞
|
| ∏ | capital pi | product – product of all values in range of series | ∏ xi=x1∙x2∙…∙xn |
| γ | Euler-Mascheroni constant |
γ = 0.527721566…
|
|
Math Symbols Name in English: Linear Algebra
All math symbols name in English related to the Linear Algebra section are discussed below.
| Linear Algebra Symbols | |||
| Math Symbol | Symbol Name | Meaning | Example |
| | A | | determinant |
determinant of matrix A
|
|
| · | dot | scalar product | a · b |
| × | cross | vector product | a × b |
| A⊗B | tensor product | tensor product of A and B | A ⊗ B |
| inner product | |||
| [ ] | brackets |
matrix of numbers
|
|
| ( ) | parentheses |
matrix of numbers
|
|
| || x || | double vertical bars | norm | |
| det(A) | determinant |
determinant of matrix A
|
|
| dim(U) | dimension | dimension of matrix A | dim(U) = 3 |
| AT | transpose | matrix transpose | (AT)ij = (A)ji |
| A† | Hermitian matrix | matrix conjugate transpose | (A†)ij = (A)ji |
| A* | Hermitian matrix | matrix conjugate transpose | (A*)ij = (A)ji |
| A -1 | inverse matrix | A A-1 = I | |
| rank(A) | matrix rank | the rank of matrix A | rank(A) = 3 |
Probability and Statistics Symbols
Statistics and probability are two distinct but connected academic areas. Probability is the likelihood that something will occur – the probability that it is that an event will occur. Probability distributions are frequently used in statistical analysis, and the two disciplines are frequently studied together. Students investigate Probability and Statistics Symbols, Naming Meanings, and Examples in the table below-
| Probability and Statistics Symbols | |||
| Math Symbols | Symbol Name | Meaning | Example |
| ∑ | summation |
summation – sum of all values in the range of series
|
|
| ∑∑ | double summation |
double summation
|
|
| P(A) | probability function | probability of event A | P(A)= 0.5 |
| P(A ∩ B) | probability of events intersection | the probability that events A and B |
P(A ∩ B)= 0.5
|
| P(A ∪ B) | probability of events union | probability that events A or B |
P(A ∪ B)= 0.5
|
| P(A | B) | conditional probability function | probability of event A given event B occurred | P(A | B)= 0.3 |
| f( X ) | probability density function (pdf) |
P( a ≤ x ≤ b ) =∫f( X ) dx
|
|
| F( X ) | cumulative distribution function (cdf) |
F( X ) =P( X ≤ x)
|
|
| μ | population mean | mean of population values | μ= 10 |
| E( X ) | expectation value | the expected value of random variable X | E( X ) = 10 |
| E( X | Y ) | conditional expectation | expected value of random variable X given Y |
E( X | Y = 2 ) = 5
|
| var( X ) | variance | variance of random variable X | var( X )= 4 |
| σ2 | variance | variance of population values | σ2= 4 |
| std( X ) | standard deviation | standard deviation of random variable X | std( X ) = 2 |
| σx | standard deviation | standard deviation value of random variable X | σx = 2 |
| median |
middle value of random variable x
|
||
| cov( X,Y ) | covariance | covariance of random variables X and Y | cov( X,Y )= 4 |
| corr( X,Y ) | correlation | correlation of random variables X and Y |
corr( X,Y )= 0.6
|
| cov( X,Y ) | covariance | covariance of random variables X and Y | cov( X,Y )= 4 |
| corr( X,Y ) | correlation | correlation of random variables X and Y |
corr( X,Y )= 0.6
|
| ρ x,y | correlation | correlation of random variables X and Y | ρ x,y= 0.6 |
| X ~ | distribution of X | distribution of random variable X | X ~ N (0,3) |
| X ~ | distribution of X | distribution of random variable X | X ~ N (0,3) |
| Mo | mode |
value that occurs most frequently in population
|
|
| MR | mid-range |
MR =( xmax+xmin)/2
|
|
| Md | sample median |
half the population is below this value
|
|
| Q1 | lower / first quartile |
25 % of population are below this value
|
|
| Q2 | median / second quartile |
50% of population are below this value = median of samples
|
|
| Q3 | upper / third quartile |
75% of population are below this value
|
|
| x | sample mean | average / arithmetic mean |
x=(2+5+9) /3=5.333
|
| s2 | sample variance | population samples variance estimator | s2= 4 |
| s | sample standard deviation | population samples standard deviation estimator | s= 2 |
| Zx | standard score | Zx=(x-x)/ Sx | |
| HG( N ,K ,n ) |
hyper-geometric distribution
|
||
| Bern( p ) |
Bernoulli distribution
|
||
| N(μσ2) | normal distribution | gaussian distribution | X ~ N (0,3) |
| U( a,b ) | uniform distribution | equal probability in range a,b | X ~ U (0,3) |
| exp(λ) | exponential distribution |
f(x)=λe-λx x≥0
|
|
| gamma(c, λ) | gamma distribution |
f(x)=λ c xc-1 e-λx / Γ ( c ) x≥0
|
|
| χ2(k) | chi-square distribution |
f(x)=xk/2-1 e-x/2 / ( 2k/2Γ )(k/2) )
|
|
| F (k1,k2) | F distribution | ||
| Bin( n,p ) | binomial distribution |
F(k) = nCk pk(1-p)n-k
|
|
| Poisson( λ ) | Poisson distribution |
F(k) = λke-λ / k !
|
|
| Geom( p ) | geometric distribution |
F(k) = p( 1-p)k
|
|
Advanced Math Symbols: Calculus
Various math symbols have appeared in calculus. All the symbols of mathematics with names and definitions can be found here. Examine all of the mathematical symbols utilized during calculus.
| Calulas Symbols | |||
| Symbol | Symbol Name in Maths | Math Symbols Meaning | Example |
| ∫ | integral | opposite to derivation |
∫xn dx = xn + 1/n + 1 + C
|
| ε | epsilon | represents a very small number, near-zero | ε → 0 |
| limx→a | limit | limit value of a function |
limx→a(3x+1)= 3 × a + 1 = 3a + 1
|
| y ‘ | derivative | derivative – Lagrange’s notation | (5×3)’ = 15×2 |
| e | e constant / Euler’s number | e = 2.718281828… |
e = lim (1+1/x)x , x→∞
|
| y(n) | nth derivative | n times derivation |
nth derivative of 3xn = 3 n (n-1)(n-2)….(2)(1)= 3n!
|
| y” | second derivative | derivative of derivative | (4×3)” = 24x |
| second derivative |
derivative of derivative
|
||
| dy/dx | derivative |
derivative – Leibniz’s notation
|
|
| nth derivative |
n times derivation
|
||
| Second derivative of time | derivative of derivative |
If y = 4t2, then
|
|
| Single derivative of time | derivative by time – Newton’s notation | y = 5t, then | |
| D2x | second derivative | derivative of derivative |
y” + 2y + 1 = 0
⇒ D2y + 2Dy + 1 = 0 |
| Dx | derivative | derivative – Euler’s notation | dy/x – 1 = 0 ⇒ Dy – 1 = 0 |
| δ | delta function |
Dirac Delta function
|
|
| partial derivative | Differentiating a function with respect to one variable considering the other variables as constant |
∂(x2+y2)/∂x = 2x
|
|
| ∭ | triple integral |
integration of the function of 3 variables
|
|
| ∬ | double integral | integration of the function of 2 variables |
∬(x3+y3)dx dy
|
| ∯ | closed surface integral | Double integral over a closed surface |
∭V (⛛.F)dV = ∯S (F.n̂) dS
|
| ∮ | closed contour / line integral | Line integral over closed curve | ∮C 2/z dz |
| [a,b] | closed interval | [a,b] = {x | a ≤ x ≤ b} | sin x ∈ [ – 1, 1] |
| ∰ | closed volume integral | Volume integral over a closed three-dimensional domain |
∰ (x2 + y2 + z2) dx dy dz
|
| (a,b) | open interval | (a,b) = {x | a < x < b} |
f is continuous within (0, 1)
|
| z* | complex conjugate | z = a+bi → z*=a-bi |
If z = 3 + 2i then z* = 3 – 2i
|
| i | imaginary unit | i ≡ √-1 | z = 3 + 2i |
| ∇ | nabla / del | gradient / divergence operator | ∇f (x,y,z) |
| vector |
A quantity with magnitude and direction
|
||
| x * y | convolution | Modification in a function due to the other function. |
y(t) = x(t) * h(t)
|
| ∞ | lemniscate | infinity symbol |
3x ≥ 0; x ∈ (0, ∞)
|
Set Theory Symbols
Set theory is a theory of mathematics that was designed to explain the groupings of items. Sets have proven to be an invaluable tool for describing some of mathematics’ most complex structures. They are primarily used to specify a wide range of real-world applications. Let us look at the many sorts of symbols employed during mathematics set theory, as well as their meanings and examples.
| Set Theory Symbols | |||
| Math Symbols | Symbol Name | Meaning | Example |
| A = B | equality | both sets have the same members | A={6,4,17}, B={6,4,17}, A=B |
| { } | set | a collection of elements | A = {3,7,9,14}, B = {9,14,28} |
| A ∩ B | intersection | objects that belong to set A and set B | A ∩ B = {9,14} |
| A ∪ B | union | objects that belong to set A or set B |
A ∪ B = {3,7,9,14,28}
|
| A ⊆ B | subset | A is a subset of B. set A is included in set B. |
{9,14,28} ⊆ {9,14,28}
|
| A ⊂ B | proper subset / strict subset | A is a subset of B, but A is not equal to B. |
{9,14} ⊂ {9,14,28}
|
| A ⊄ B | not subset | set A is not a subset of set B |
{9,66} ⊄ {9,14,28}
|
| A ⊇ B | superset | A is a superset of B. set A includes set B |
{9,14,28} ⊇ {9,14,28}
|
| A ⊃ B | proper superset / strict superset | A is a superset of B, but B is not equal to A. |
{9,14,28} ⊃ {9,14}
|
| A ⊅ B | not superset | set A is not a superset of set B |
{9,14,28} ⊅ {9,66}
|
| 2A | power set |
all subsets of A
|
|
| power set |
all subsets of A
|
||
| A×B | cartesian product | set of all ordered pairs from A and B |
A×B = {(a,b)|a∈A , b∈B}
|
| Ac | complement |
all the objects that do not belong to set A
|
|
| A \ B | relative complement | objects that belong to A and not to B | A = {3,9,14}, B = {1,2,3}, A-B = {9,14} |
| A – B | relative complement | objects that belong to A and not to B | A = {3,9,14}, B = {1,2,3}, A-B = {9,14} |
| A ∆ B | symmetric difference | objects that belong to A or B but not to their intersection |
A = {3,9,14},
B = {1,2,3}, A ∆ B = {1,2,9,14} |
| A ⊖ B | symmetric difference | objects that belong to A or B but not to their intersection |
A = {3,9,14},
B = {1,2,3}, A ⊖ B = {1,2,9,14} |
| a∈A | element of, belongs to |
set membership |
A={3,9,14}, 3 ∈ A
|
| x∉A | not element of | no set membership |
A={3,9,14}, 1 ∉ A
|
| (a,b) | ordered pair |
collection of 2 elements
|
|
|
complex numbers set
|
6+2i ∈ | ||
| |A| | cardinality | the number of elements of set A |
A={3,9,14}, |A|=3
|
| #A | cardinality | the number of elements of set A |
A={3,9,14}, #A=3
|
| | | vertical bar | such that | A={x|3<x<14} |
| aleph-null |
infinite cardinality of natural numbers set
|
||
| aleph-one |
cardinality of countable ordinal numbers set
|
||
| Ø | empty set | Ø = { } | C = {Ø} |
| universal set |
set of all possible values
|
||
| 0 | natural numbers / whole numbers set (with zero) | 0 = {0,1,2,3,4,…} | 0 ∈ 0 |
| 1 | natural numbers / whole numbers set (without zero) | 1 = {1,2,3,4,5,…} | 6 ∈ 1 |
|
integer numbers set
|
-6 ∈ | ||
|
rational numbers set
|
2/6 ∈ | ||
|
real numbers set
|
6.343434∈ | ||
Mathematical Language and Symbols
Roman numerals are employed in a variety of applications and are frequently seen in our daily lives. The followings are the most popular Roman and European numeric symbols used in mathematics.
| Numerical Symbols | ||
| Name | European | Roman |
| zero | 0 | n/a |
| one | 1 | I |
| two | 2 | II |
| three | 3 | III |
| four | 4 | IV |
| five | 5 | V |
| six | 6 | VI |
| seven | 7 | VII |
| eight | 8 | VIII |
| nine | 9 | IX |
| ten | 10 | X |
| eleven | 11 | XI |
| twelve | 12 | XII |
| thirteen | 13 | XIII |
| fourteen | 14 | XIV |
| fifteen | 15 | XV |
| sixteen | 16 | XVI |
| seventeen | 17 | XVII |
| eighteen | 18 | XVIII |
| nineteen | 19 | XIX |
| twenty | 20 | XX |
| thirty | 30 | XXX |
| forty | 40 | XL |
| fifty | 50 | L |
| sixty | 60 | LX |
| seventy | 70 | LXX |
| eighty | 80 | LXXX |
| ninety | 90 | XC |
| one hundred | 100 | C |








 d -and f -Block Elements NEET Notes, Che...
d -and f -Block Elements NEET Notes, Che...
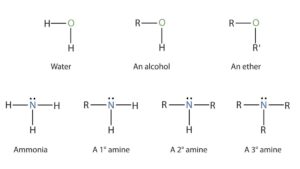 NEET 2026 Amines Chapter Notes: Key Topi...
NEET 2026 Amines Chapter Notes: Key Topi...
 Electrochemistry NEET Notes, Check Impor...
Electrochemistry NEET Notes, Check Impor...












