What is the difference between Natural numbers and whole numbers?

The Main differences between Natural numbers and whole numbers are pointed out below - • The range of whole numbers is zero to infinity. Numbers from 1 to infinity are referred to as "natural numbers." W = {0,1,2,3,4,5,6,7,8,9,10...∞} N = {1,2,3,4,5,6,7,8,9,10...∞} • Whole numbers include 0 (zero), while natural numbers do not. Whole Numbers are not a subset of Natural Numbers, but Natural Numbers are a subset of Whole Numbers.

Natural Numbers List, Definition and Examples, Class 10 & 11

Table of Contents

Natural Numbers

As we know Number system is the fundamental stone of Mathematics. Natural Number is a part of the Number system. Positive numbers from 1 to infinity make up Natural Numbers, it does not contain zero and negative integers. Natural numbers are also known as counting numbers Because we use natural numbers while counting something. In this article, we will get a clear and detailed analysis of Natural Numbers and solve some numerical problems.

Natural Numbers Definition and Examples

The collection of numbers known as “Natural numbers” is made up of positive integers or numbers from 1 to infinity. Natural numbers do not include 0, negative integers (such as -1, -35, etc.), fractions, or decimals.
These natural numbers are often used while counting anything, transacting money, or determining temperature, time, or amount; thus, they are also known as counting numbers. 3 bicycles, 5 buses, etc.
Natural numbers from 1 to 10 are: 1, 2, 3, 5, 6, 7, 8, 9, 10.

Natural numbers” is made up of positive integers or numbers from 1 to infinity.Some examples of Natural Numbers are 1,10, 45, 29,99,275,360 ,30000,1538296 and so on.

Natural Numbers kya hote hai in Hindi

“प्राकृतिक संख्या” के रूप में जानी जाने वाली संख्याओं का संग्रह धनात्मक पूर्णांकों या 1 से अनंत तक की संख्याओं से बना होता है। प्राकृत संख्याओं में 0, ऋणात्मक पूर्णांक (जैसे -1, -35, आदि), भिन्न या दशमलव शामिल नहीं हैं।
इन प्राकृतिक संख्याओं का उपयोग अक्सर कुछ भी गिनते समय, पैसे का लेन-देन करते समय, या तापमान, समय, या राशि का निर्धारण करते समय किया जाता है; इस प्रकार, उन्हें गिनती संख्या के रूप में भी जाना जाता है। 3 साइकिलें, 5 बसें, आदि।
1 से 10 तक की प्राकृत संख्याएँ हैं: 1, 2, 3, 5, 6, 7, 8, 9, 10।

Natural Numbers Sum

Numbers	Sum
1-10	55
1-100	5050
1-1000	500500
1-10000	50005000
1-100000	5000050000
1-1000000	500000500000

Sum of n Natural Numbers

For AP of natural numbers, a = 1 and d = 1, the Sum of n terms S_n of this AP can be found using the formula-

Sn = n/2[2×1+(n-1)1]

S_n = n(n+1)/2

Smallest Natural Number

The smallest Natural Number is Zero [ 0 ].

Natural Numbers List From 1 to 50

Natural integers between 1 and 50 are 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12, 13, 14, 15, 16, 17, 18, 19, 20, 21, 22, 23, 24, 25, 26, 27, 28, 29, 30, 31, 32, 33, 34, 35, 36, 37, 38, 39, 40, 41, 42, 43, 44, 45, 46, 47, 48, 49,and 50

What are Natural Numbers Symbol?

A set of Natural Numbers defines as a collection of natural numbers. In the field of Mathematics set of natural numbers is represented by N and written as {1,2,3,…….}.
Thus, the Set of Natural Numbers is
N = {1,2,3,4,5,6,7,8,9,10…∞}

A group of Natural Numbers can be expressed in a variety of ways. Such as,
N = Set of all numbers starting from 1 to infinity (∞) [Statement Form]
N = {1, 2, 3, 4, 5, 6, 7, 8, 9, 10,……,) [Roaster Form]
N = {x : x is an integer starting from 1} [Set Builder Form]

Natural Numbers Odd Number set

Numbers that are divisible only by one and themselves and can’t be divided into two equal parts are called Odd Numbers. Set of Natural Odd Numbers is N ={ 1,3,5,7,9,11,13, ……….}

Natural Numbers Even Number set

Numbers that are divisible by 2 and can be divided into two equal parts are called Even Numbers.Set of Natural Odd Numbers is N ={ 2,4,6,8,10,12,14, ……….}

All Natural Numbers are Whole Numbers

Yes, All the natural numbers are also whole numbers.

Natural Numbers are Whole Numbers: Difference

Before We know about the differences between whole numbers and Natural numbers. First, Let’s what is a whole number.
The set of natural numbers, which includes zero, is referred to as the set of whole numbers in the number system. The set of whole numbers in mathematics is represented by the symbol W, which is written as { 0, 1, 2, 3, …….}
W = {0,1,2,3,4,5,6,7,8,9,10…∞}
Set of Natural Numbers is
N = {1,2,3,4,5,6,7,8,9,10…∞}

The Main differences between Natural numbers and whole numbers are pointed out below –

Natural Number	Whole Number
The range of whole numbers is zero to infinity. W = {0,1,2,3,4,5,6,7,8,9,10…∞}	Numbers from 1 to infinity are referred to as “natural numbers.” N = {1,2,3,4,5,6,7,8,9,10…∞}
2)Natural numbers do not include 0 (Zero)	2) Whole numbers include 0 (zero)
3)Natural Numbers are a subset of Whole Numbers.	3) But Whole Numbers are not a subset of Natural Numbers,

Natural numbers are a subset of whole numbers-Is it True?

Yes. It is possible to define Natural Number as a subset of a Whole Number. because the entire set of numbers runs from 0 to infinity. As opposed to the Natural Number Set, which runs from 1 to infinity. It is simple to state that the whole number contains all natural numbers. Natural numbers are therefore a subset of the entire set of numbers.

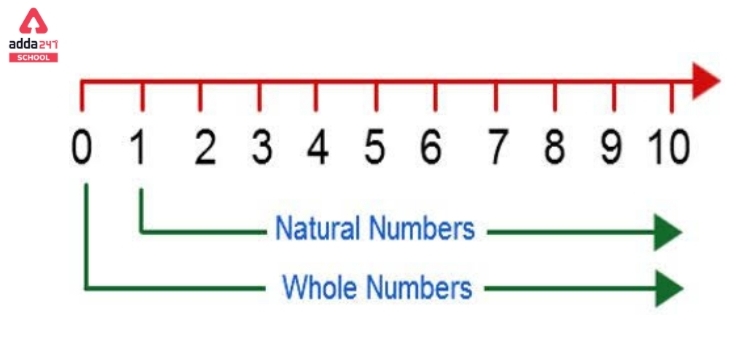
Natural Numbers Start from in Number Line

Natural Numbers are all positive numbers from 1 to infinity; they do not include zero or negative integers.On a number line, natural numbers are represented as follows:

Natural Numbers List, Definition and Examples, Class 10 & 11_3.1 — Natural Numbers on Number Line

Zero: Is it a Natural Number?

No. Zero is not a Natural Number .it is a whole Number. The Value of Zero is null. Natural Number set starts from 1 to infinity. As we know Natural numbers are also called Counting Numbers because we use these numbers while counting. We Can’t use Zero in counting.
Zero is a part of the whole Number but not a natural number.

Natural Numbers Starts from

Natural numbers starts from 1 and continue indefinitely. They are a set of positive whole numbers that do not include zero or any negative numbers. So, the sequence of natural numbers begins with 1 and goes on as follows: 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, and so forth, with no end in sight.