The NCERT class 12 chemistry chapter 4 discusses the characteristics of transition metals, coloured ions and complex compounds, variations in element sizes, ionization energies, magnetic features, and oxidation states. The chapter addresses d-block elements along with their characteristics and variations.
Class 12 Chemistry NCERT Solutions includes various significant subjects. Students are strongly advised to review every topic in depth to achieve a thorough understanding of the concepts presented in the chapter and to utilize the given solutions effectively.
Class 12 Chemistry Chapter 4 NCERT Solutions
This chapter deals with the elements of D and F blocks in the table. These NCERT solutions stem from the committed efforts of the Adda247 teachers focused on helping students understand the concepts presented in this chapter. Through reviewing and practising these solutions, the goal is for students to effortlessly attain outstanding results in their examinations.
The d-block of the periodic table contains elements of groups 3-12 in which the d orbitals are progressively filled in each of the four long periods. The f – f-block consists of elements in which 4 f and 5 f orbitals are progressively filled. They are placed in a separate panel at the bottom of the periodic table.
Characteristics of d and f block Elements.
- These are metallic in nature.
- They are hard and have high densities.
- They have high melting and boiling point.
- They shows variable oxidation states.
- They form coloured ions and compounds.
- The atomic radii decrease with increase in atomic number.
The main difference between d-block elements and f-block elements is that d-block elements are chemical elements having electrons filled to their d orbitals whereas f-block elements are chemical elements having electrons filled to their f orbitals.
Class 12 Chemistry Chapter 4 NCERT Solutions
Q) To what extent do the electronic configuration decide the stability of oxidation states in the first series of the transition elements? Illustrate your answer with examples.
Answer: The elements in the first half of the transition series exhibit many oxidation states with Mn exhibiting maximum no. of oxidation state (+2 to +7). The stability of +2 oxidation state increases with the increase in atomic no. This happens as more electrons are getting filled in the d orbital. However, Sc does not show a +2 oxidation state. Its electronic configuration is 4s23d1. It loses all the 3 electrons to form Sc3+. +3 oxidation state of Sc is very stable as by losing all three electrons, it attains stable noble gas configuration, [Ar]. Ti(+4) and V(5) are very stable for the same reason. For Mn, the +2 oxidation state is very stable as after losing two electrons, its d orbital is exactly half-filled, [Ar]3d5.
Q) What may be the stable oxidation state of the transition element with the following d elements d electron configuration in the ground state of their atoms?
Answer:
| Electronic configuration in ground state | Stable oxidation state |
| 3d3 (Vanadium) | +2, +3, +4, +5 |
| 3d5 (chromium) | +3, +4, +6 |
| 3d5 (Manganese) | +2, +4, +6, +7 |
| 3d8(coblt) | +2, +3 |
| 3d4 | There is no 3d4 configuration in ground state |
Q) Name the oxometal anions of the first series of the transition element with the following d electron configurations in the ground state of their atoms: 3d3, 3d5.3d8 and 3d4?
Answer:
| Name of oxometal anion | Name of metal with oxidation state | Group no. to which metal belong |
| CrO42-
MnO4– |
Cr in +6 state of oxidation
Mn in +7 state |
6th group
7th group |
| Vanadate | Oxidation state +5 |
Q) What is lanthanoid contraction? What are the consequences of lanthanoid contraction?
Answer: The steady decrease in the size of lanthanoid ions (M3+) with the increase in atomic number is called lanthanoid contraction.
Consequences of lanthanoid contraction :
Due to lanthanoid contraction, the size of M3+ ions decreases and there is increase in the covalent character in M – OH bond.
Q) In what way is the electronic configuration of the transition elements different from that of the non-transition elements?
Answer: Transition elements have partially filled d orbital but the non-transition elements have no d orbital or have completely filled d orbitals.
Q) What are the characteristics of the transition elements and why are they called transition elements? Which of the d block element may not be regarded as the transition element?
Answer: Transition elements are those elements in which the atoms or ions (in stable oxidation state) contain partially filled d-orbital. These elements lie in the d-block and show a transition of properties between s-block and p-block. Therefore, these are called transition elements.
Elements such as Zn, Cd, and Hg cannot be classified as transition elements because these have completely filled d-subshell.
Q) Explain giving reasons:
- Transition metals and many of their compounds show paramagnetic behaviour.
- The enthalpies of atomisation of the transition metals are high.
- The transition metals generally form coloured compounds.
- Transition metals and their many compounds act as good catalyst.
Answer:
i.)Transition metals show paramagnetic behaviour. Paramagnetism arises due to the presence of unpaired electrons with each electron having a magnetic moment associated with its spin angular momentum and orbital angular momentum. However, in the first transition series, the orbital angular momentum is quenched. Therefore, the resulting paramagnetism is only because of the unpaired electron.
(ii) Transition elements have high effective nuclear charge and a large number of valence electrons. Therefore, they form very strong metallic bonds. As a result, the enthalpy of atomization of transition metals is high.
(iii) Most of the complexes of transition metals are coloured. This is because of the absorption of radiation from visible light region to promote an electron from one of the d-orbitals to another. In the presence of ligands, the d-orbitals split up into two sets of orbitals having different energies. Therefore, the transition of electrons can take place from one set to another. The energy required for these transitions is quite small and falls in the visible region of radiation. The ions of transition metals absorb the radiation of a particular wavelength and the rest is reflected, imparting colour to the solution.
(iv) The catalytic activity of the transition elements can be explained by two basic facts.
(a) Owing to their ability to show variable oxidation states and form complexes, transition metals form unstable intermediate compounds. Thus, they provide a new path with lower activation energy, Ea, for the reaction.
(b) Transition metals also provide a suitable surface for the reactions to occur.
Q) What are the interstitial compounds? Why are such compounds well known for transition metals?
Answer: The compounds formed when small atoms of H, C or N get trapped inside the crystal lattice of metals is known as interstitial compounds. A number of interstitial compounds are formed by the transition metals. As vacant spaces of the transition metals are filled up by small atoms, these compounds are hard and rigid.
Interstitial compounds are well known for transition compounds due to its closed crystalline structure with voids in them. The atomic size of transition metals are very large hence have large voids to occupy these small atoms.
Q) How is the variability in oxidation states of transition metals different from that of the non-transition metals? Illustrate with example.
Answer:
The non-transition elements also show variable oxidation states. However these differ from the variable oxidation states shown by transition elements. Variable oxidation states shown by transition elements can differ by one unit while the oxidation states shown by non-transition elements differ by 2 unit due to inert pair effect.
For example, thallium exhibits oxidation states +1, +3 and lead exhibits +2, +4 oxidation states. Moreover, in case of transition elements of the same group, higher oxidation state is more stable for heavier elements. e.g., Mo (VI) and W (VI) are more stable than Cr (VI). In non-transition elements of p-block, lower oxidation state is more stable due to inert pair effect e.g., Pb2+ is more stable than Pb4+.
Q) Describe the preparation of potassium dichromate from iron chromite ore. What is the effect of increasing pH on a solution of potassium dichromate?
Answer: Potassium dichromate is prepared from chromate in the following steps:
Step 1: Preparation of sodium chromate
4FeCr2O7 + 16NaOH + 7O2 -à 8NaCrO4 +2Fe2O3 +8H2O
Step 2: Conversion of sodium chromate into sodium dichromate
2Na2Cro4 + Conc.H2SO4 -à Na2Cr2O7 + Na2SO4 + H2O
Step 3: Conversion of sodium dichromate to potassium dichromate
Na2Cr2O7 + 2KCl à K2Cr2O7 + 2NaCl
Potassium dichromate being less soluble than the sodium chloride is obtained in the form of crystals and can be removed.
The dichromate ion exist in equilibrium with chromate ion at pH and however, by changing the pH, they can be interconverted.
Q) Explain why Cu+ ion is not stable in aqueous solutions?
Answer: Cu+ (aq) is not stable, while Cu2+ (aq) is stable. This is becuase ΔhydH of Cu2+(aq) is much higher than that of Cu+(aq) and hence it compensates for the 2nd IE of Cu. Thus, many Cu(I) compounds are unstable in aqueous solution and undergo disproportionation as follows :
2 Cu+ —–> Cu2+ + Cu
Q) What are the characteristics of the transition . elements and why are they called transition elements? Which of the d-block elements may not be regarded as the transition elements?
Answer: General characteristics of transition elements.
(i)Electronic configuration – (n -1) d1-10 ns1-2
(ii)Metallic character – With the exceptions of Zn, Cd and Hg, they have typical metallic structures.
(iii)Atomic and ionic size-ions of same charge in a given series show progressive decrease in radius with increasing atomic number.
(iv)Oxidation state-Variable; ranging from+2 to +7.
(v)Paramagnetism – The ions with unpaired electrons are paramagnetic.
(vi)Ionisation enthalpy – Increases with increase in charge.
Formation of coloured ions – Due to presence of unpaired electrons.
(viii) Formation of complex compounds – Due to small size and high charge density of metal ions.
(ix)They possess catalj^c properties – Due to
their ability to adopt multiple oxidation states. .
(x)Formation of interstitial compounds.
(xi)Alloy formation.
They are called transition elements due to their incompletely filled d-orbitals in ground state or in any stable oxidation state and they are placed between s and p- block elements. Zn, Cd and Hg have fully filled d- orbitals in their ground state hence may not be regarded as the transition elements.
Q) Actinoid contraction is greater from element to element than lanthanoid contraction. Why?
Answer: The decrease or contraction in atomic radii, as well as ionic radii in actinoid elements (actinoid contraction), is more as compared to lanthanoid contraction because 5/ electrons have more poor shielding effect as compared to 4f electrons. Therefore, the effect of increased nuclear charge leading to contraction in size is more in case of actinoid elements.
Q) Describe the oxidising action of potassium dichromate and write the ionic equations for its reaction with:
(i)iodide
(ii)iron (II) solution and
(iii)H2S
Answer: K2Gr207is a powerful oxidising agent. In dilute sulphuric acid medium the oxidation state of Cr changes from+6 to + 3. The oxidising action can be represented as follows:
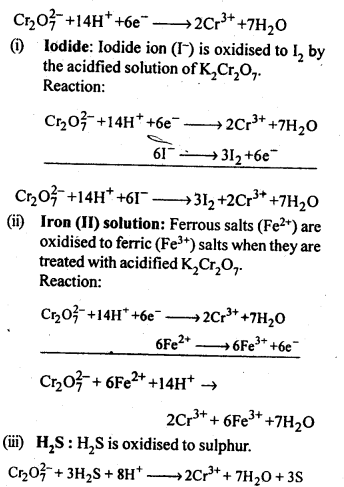
Q) Compare the stability of +2 oxidation state for the elements of the first transition series.










 CBSE Class 12th Business Studies Case Ba...
CBSE Class 12th Business Studies Case Ba...
 Photosynthesis in Higher Plants Biology ...
Photosynthesis in Higher Plants Biology ...
 AILET Sample Paper 2026 Out, Download PD...
AILET Sample Paper 2026 Out, Download PD...

