Percentage Error Formula and Questions with Examples -

Table of Contents

Define Percent Error

Percent error is the difference between something’s actual or known value and its approximate or measured value. It is used to report the difference between the experimental value and its real or exact value in scientific experiments. It’s estimated as a percentage of the actual values. As an example, if you look at a gumball machine and estimate how many gumballs are in there, and then go ahead and calculate the number of gumballs, you will be able to calculate the percent mistake you made in your guess. In this article, we have discussed percent error, we have given all the information in laymen’s language so students facing issues in percent error can understand the concept. Bookmark this page to get all the related topics. know Percentage Error Formula in this post.

Read: Velocity Formula

Percent Error Formula in Physics

The difference between a measured and exact value, divided by the known value, and multiplied by 100 is the percentage error. Percentage error is often expressed as a positive number in many applications. Normally, the error’s absolute value is divided by an accepted value and expressed in percentage.

Absolute Difference = Accepted Value – Experimental Value

Percent Error = (Absolute Difference/ Accepted Value)* 100

The absolute value is usually taken as the experimental or measured value minus the known or theoretical value. As a result, the Percent Error Formula will be

Percent Error = (Experimental value- theoretical value/ theoretical value)*100

If your percentage error is close to zero, your approximation is very close to the true value. This formula is essential in determining the accuracy of your calculations. The percent error is usually expressed as a positive number in most applications, but in some areas, such as chemistry, it is conventional to write it as a negative number because a positive value in chemistry would indicate a potential problem with the experiment or unaccounted for reactions.

Percent Error Formula in Maths

The percentage error formula is used to calculate the relative error or the accuracy of a measured or experimental value compared to the accepted or theoretical value. It is expressed as a percentage and is given by the following formula:

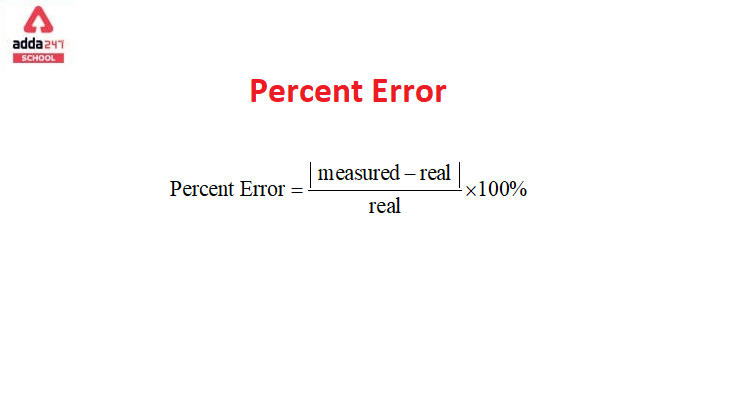
Percentage Error = [(|Measured Value – Accepted Value|) / |Accepted Value|] * 100

Where:

“Measured Value” is the value obtained from a measurement or an experiment.
“Accepted Value” is the true or theoretical value, often obtained from reliable references or previously established data.

To calculate the percentage error, you take the absolute difference between the measured value and the accepted value, divide it by the absolute value of the accepted value, and then multiply by 100 to express the result as a percentage.

The percentage error indicates how much the measured value deviates from the accepted value, allowing for an assessment of the accuracy of the measurement or experiment. A percentage error of zero indicates a perfect match between the measured and accepted values, while a nonzero percentage error shows the degree of deviation. Negative values are possible and typically indicate that the measured value is lower than the accepted value, while positive values indicate that the measured value is higher than the accepted value.

Read: Digestive System of Human

How to Calculate the Percentage Error with Example?

Here we have given some steps to calculate the percentage error, students can follow the steps to calculate the percentage error.

Subtracting one value from another provides the “error” value. If you’re not keeping the sign, the sequence doesn’t matter; however, if you’re keeping a negative sign, you’ll need to deduct the precise value from the measured value to get the “error” value.
The “error” value is then divided by the known or accurate value. You’ll get a decimal number from this division.
To convert this decimal value to a percent value multiply the number by 100.
Finally, to reflect your percent error, you would use a percent sign in front of the calculated value.

Example: Ramesh got a traffic penalty notice for police speeding for traveling 70 mph in a 60 mph zone. Ramesh claimed his speedometer said 60 mph, not 70 mph. What could Ramesh claim as his percent error?

Solution:

Let us arrive at %error in 3 steps:

Absolute Error = |70−60| = 10

Percent Error = 10/60=0.1667

= 0.1667×100 = 16.67%

Ramesh can claim 16.67% as his percent error

Percent Error Solved Question with Example

Here we have given some solved examples of percent error, the students must practice the questions from percent error instead of mugging up the concept. To ease students, we have given the example, the students can refer to these examples. Many students struggled in percent error because they just try to mug up the concepts and ignore the question-solving part, application is more important than mugging concept. Refer to the questions and practice the similar patterns of questions to consolidate the percent error topic.

Q. I estimated that 60 people would attend the show. However, just 70 people attended the performance on the day of the event. Find out how much of my calculation was off by a percentage.

Solution: We’ll start by calculating the absolute difference of values . which is

Absolute Difference = Accepted Value – Experimental Value

Absolute Difference = 60-70 = 10

we will use the percent error formula to calculate the error.

Percentage Error = (Absolute Difference/ Accepted Value)* 100

Percentage Error = (10/70)*100

PercentageError = 14.28

Here the percentage error is 14.28.

Q. Due to some minor inaccuracies, a scale incorrectly measures a value of 12 cm. If the actual measurement of the value is 10 cm, calculate the percentage inaccuracy.

Solution: Based on the information provided in the problem,

12 cm is the experimental value.

8 cm is the theoretical value.

Using the formula to calculate the result,

Percentage Error = (Experimental value- theoretical value/ theoretical value)*100

Percentage Error = (12-8/8)*100

Percentage Error = 50%

Percentage The error rate was calculated to be 50%.

FAQs

Q. How do you calculate the percent error?

Percent error is the difference between something's actual or known value and its approximate or measured value. The formula to calculate percent error is

Absolute Difference = Accepted Value - Experimental Value

Percentage Error = (Absolute Difference/ Accepted Value)* 100

Q. How do you interpret percent error?

When you measure something in an experiment, percent errors tell you how big your error is. Smaller values indicate that you are near the acceptable or true value. A 0.5 percent error, for example, indicates that you were extremely close to the true value, whereas a 50 percent error indicates that you were very far from the true value.

Q. What is a good percent error?

In some cases, 8% error is acceptable, or even higher errors are acceptable but in some cases, even 0.5% error may be too high. To reach one solution, many high school and university professors came to the conclusion that a 5% error will be acceptable.

Q. Why percent error is important?

Mathematicians and scientists are interested in seeing how well theoretical concepts match actual results. They can utilise the percent error to figure out how what actually happened and what they thought happened are related.