Real Numbers Class 10 Notes, MCQs, Extra Questions

Real Numbers Class 10 Notes

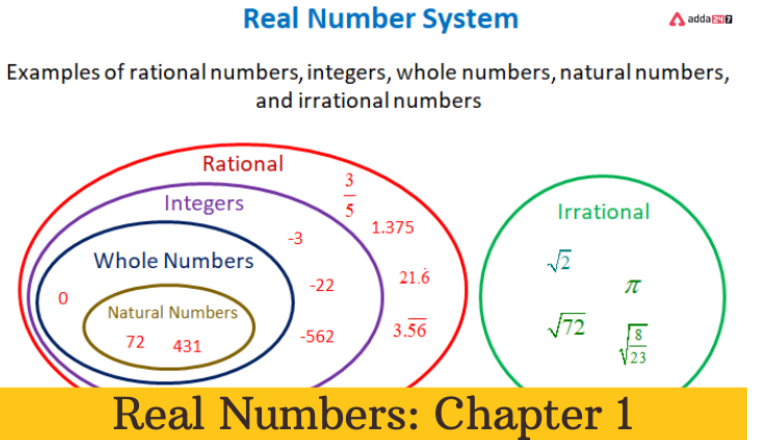
R= Real Numbers

Real numbers include both irrational and rational numbers.

I= Integers

Integers are all numbers starting with (…-3, -2, -1, 0, 1, 2, 3…).

Q= Rational Numbers

Rational numbers are real numbers with the pattern p/q, q 0, and p, q I.

• Rational expressions can represent all integers, such as 5 = 5/1.
• Decimal expansion of recurring or non-recurring rational numbers.

Q’ = Irrational Numbers

Real numbers cannot be expressed in the form p/q, whose decimal expansions are non-terminating and non-recurring.

• Roots of primes like √2, √3, √5 etc., are irrational.

N= Natural Numbers

Natural numbers are those that can be counted. N = {1, 2, 3, …}

W= Whole Numbers

Whole numbers are the collective term for zero and all natural numbers. {0, 1, 2, 3,…}

Even Numbers

Even numbers are natural numbers with the form 2n. (2, 4, 6, …}

Odd Numbers

Odd numbers are natural numbers with the form 2n – 1; examples are 1, 3, 5, etc.

• Why can’t the form be expressed as 2n+1?

Remember!

*Natural numbers are exclusively whole numbers.

*Every Whole Number is an integer.

*Rational Numbers are the same as Integers.

*All Rational Numbers are Real Numbers.

Prime Numbers

Prime numbers are all natural numbers bigger than one that can be divided by 1 and the number itself. Examples of prime numbers are 2, 3, 5, 7, and 11.

• Since it only has one factor, 1, it is not a prime number.

Composite Numbers

Composite numbers are any natural numbers that may be divided by one, including the number one itself. Four, six, eight, nine, ten, etc.

• 1 is not a prime number nor a composite number.

Real Numbers Class 10 Notes- Methods

1. Euclid’s Division Lemma

There are distinct integers q and r that satisfy the equation a = bq + r, 0 r b when given two positive integers a and b.

Observe this: Every time, “r” is lower than “b.” Each “q” and “r” is distinctive.

1. Application Of Lemma

The HCF of two positive numbers is determined using Euclid’s Division Lemma. 

Example: How can I find the HCF of 56 and 72?

Steps:- 

• Lemma applied to 56 and 72.
• Find “b” and “r” by using a larger number. 72 = 56 × 1 + 16
• Consider 56 as the new dividend and 16 as the new divisor since 16 s not equal to 0. 56 = 16 × 3 + 8
• Once more, if 8 is not equal to 0, apply 16 as the new dividend and 8 as the new divisor. 16 = 8 × 2 + 0

The remainder being zero, (8) is HCF’s divisor

Euclid’s Division Lemma can be extended to all integers except zero, i.e., b 0. This is true even if it is only stated for positive integers.

III. Constructing A Factor Tree

Steps for building a factor tree:

• Put the amount in the form of a prime number plus a composite number.

Example: 48 factorise

• Continue until all primes have been achieved. 

∴ Prime factorization of 48 = 24 x 3

1. Fundamental Theorem Of Arithmetic

With the exception of the sequence in which they appear, every composite number can be written as a product of primes, and this expression is singular.

Applications:

• In order to find the HCF and LCM of two or more positive integers.
• Demonstrating the irrationality of numbers
• To identify the type of rational number’s decimal expansion.

1. Finding The HCF And LCM Of Two Or More Positive Numbers Using An Algorithm

Step I: The first step is to factorise each of the positive integers that are given and express each as a product of powers of primes that are arranged in ascending order of prime number.

Step II: To determine HCF, locate the least powerful prime factors and multiply them to obtain HCF.

Step III: Find the largest exponent and multiply it by the exponents to determine the LCM.

2. Demonstrating The Irrationality Of Numbers

• Irrational numbers are those whose sum or difference is not rational.
• An irrational number is the product of a non-zero rational number, or its quotient.

3. To Ascertain How Rational Numbers Expand To Decimal Places:

• Let x = p/q, where p and q are co-primes, represent a rational number with an end to its decimal expansion. When m and n are non-negative integers, the prime factorization of “q” takes the form 2m5n.
• If x = p/q is a rational number with p and q being non-negative integers, and q’s prime factorization does not take the form 2m5n, then x has a non-terminating repeated decimal expansion.

Alert!

• 23 can be written as: 23 = 2350
• 52 can be written as: 52 = 2052

Real Numbers For Class 10 Extra MCQ Questions

Ques. The number 7×11×13×15+15 is a

(a) Composite Number

(b) Whole Number

(c) Prime Number

(d) None of these

Ans: (a) and (b) both

Ques. A lemma is an axiom used for providing

(a) other statement

(b) no statement

(c) contradictory statement

(d) none of these

Ans: (a) other statement

Ques. Given that HCF (306,657)=9, find LCM (306,657).                  

Ans: It is given that the HCF of the two numbers 306,657 is 9. We have to find its LCM.

We know that, LCM×HCF = Product of two numbers. Therefore, LCM×HCF= 306×657 

⇒LCM= 306×657/HCF

⇒LCM= 306×657/9 

∴LCM=22338

Ques. Prove that 3 + 2√5 is irrational.

Ans: Let 3 + 2√5 be a rational number.

Then the co-primes x and y of the given rational number where (y ≠ 0) is such that:

3 + 2√5 = x/y

Rearranging, we get,

2√5 = (x/y) – 3

√5 = 1/2[(x/y) – 3]

Since x and y are integers, thus, 1/2[(x/y) – 3] is a rational number.

Therefore, √5 is also a rational number. But this confronts the fact that √5 is irrational.

Thus, our assumption that 3 + 2√5 is a rational number is wrong.

Hence, 3 + 2√5 is irrational.

Ques. Check whether 6n can end with the digit 0 for any natural number n.

Ans: If the number 6n ends with the digit zero (0), then it should be divisible by 5, as we know any number with a unit place as 0 or 5 is divisible by 5.

Prime factorization of 6n = (2 × 3)n

Therefore, the prime factorization of 6n doesn’t contain the prime number 5.

Hence, it is clear that for any natural number n, 6n is not divisible by 5 and thus it proves that 6n cannot end with the digit 0 for any natural number n.

Ques. Using Euclid’s Algorithm, find the HCF of 2048 and 960.

Ans. 2048 > 960

Using Euclid’s division algorithm,

2048 = 960 × 2 + 128

960 = 128 × 7 + 64

128 = 64 × 2 + 0

Therefore, the HCF of 2048 and 960 is 64.

Sharing is caring!