Spring Constant- Definition, Formula, Value, Examples

Springs are used frequently in our day-to-day lives. Some springs are stiffer than others due to the uniqueness of the spring known as the spring constant. This constant determines the stiffness or elasticity property of a spring. It is described as the amount of force needed to stretch or compress a spring a unit distance. The constant of the spring gives a unique ability to the spring and tells about the elasticity of the spring.

Spring Constant Definition

The spring constant of a spring is an important concept related to SHM (Simple Harmonic Motion). It is one of the key concepts in Hooke’s law and is used in determining the elasticity of an object. In simple words, the constant factor of the spring is equal to the force applied when the displacement in the string is 1 unit. Formally, this constant is denoted by K. Let us now delve into various concepts related to this special constant.

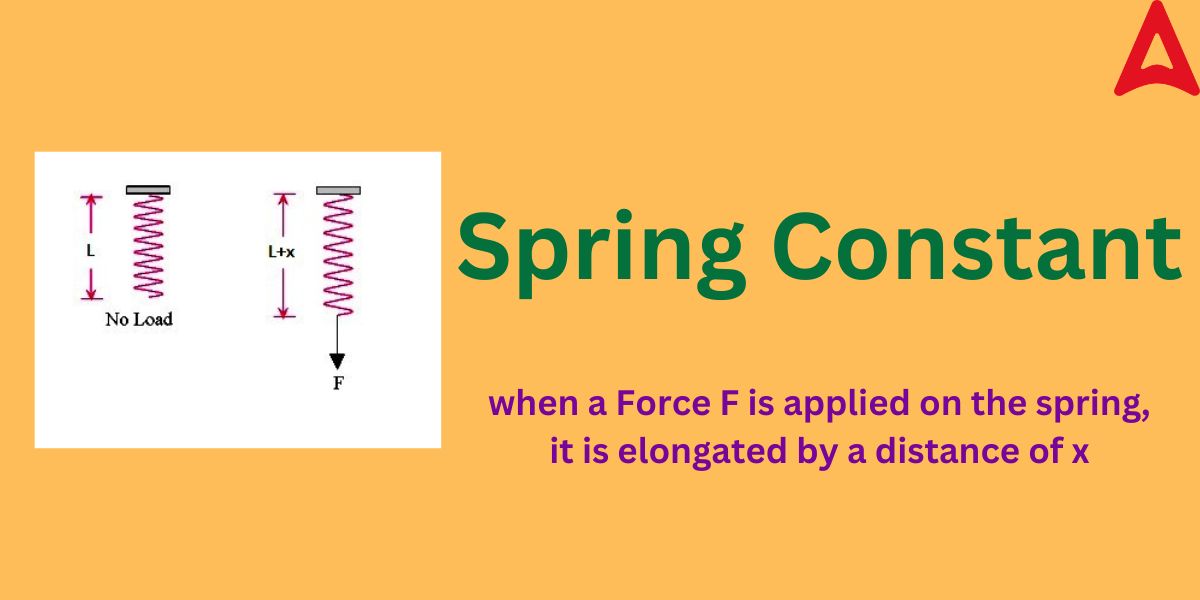
Spring Constant Formula

The formula of the spring constant is derived using Hooke’s Law. As per Hooke’s Law, the force needed to stretch or compress a spring is directly proportional to the length by which the spring is compressed or stretched. So using this law, we get:

F ∝ x

where, x is the length by which the spring is compressed or stretched. 

F = Forced applied

By removing the proportionality sign, we get

F = -kx

where , k = spring’s constant

The negative (-) sign denotes that the force that is restoring the original position is opposite to the direction of stretching or compression. 

The formula can also be written as:

k = -F/x

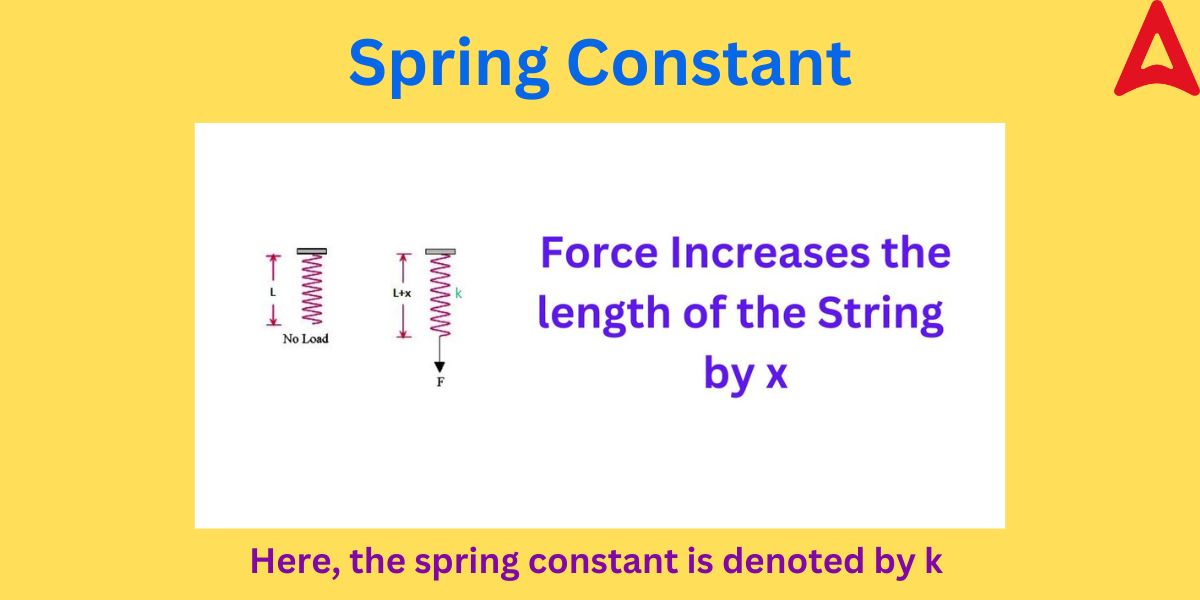
Spring Constant Units

Like every other physical quantity, the constant for the spring too possesses an SI unit. Let us derive the SI unit of the spring constant using the basic rule of units. 

As we know, k = – F/x

Putting the SI units of the known physical quantity and ignoring the negative sign, we get:

k = N/m

So, the SI unit of this constant is N/m.

Spring Constant Mass Formula

The constant for a spring can be related to the mass of the object. The spring constant formula with mass is given below.

As F = -kx

We know that F = ma, where m is the mass and a is the acceleration

So, ma = -kx

Or, k = -ma/x

Spring Constant Value

For any material in the world, the value of its spring’s constant factor is always positive. It must also be noted that this constant value is always constant for a given spring and it is independent of the magnitude of force applied. However, this constant value changes with the length of the spring. The spring’s constant of a given spring is inversely proportional to the linear dimension, i.e., the length of the spring. 

K ∝ 1/L

Where, K is the spring’s constant and L is the length of the spring.

Spring Constant Dimensional Formula

We can determine the dimensional formula of the constant of a spring by the following method.

As we know F = – kx

Putting the dimensions of Force and Length in the above equation:

[MLT^-2] = -k [L]

=> -k = [MLT^-2]/[L]

=> k = –[MT^-2]

we can ignore the negative (-) sign, so, the dimension formula becomes [MT^-2]

Spring Constant and SHM

According to Newton’s third law of motion, when the spring is pulled, an equal and opposite force is applied in the opposite direction. This helps the spring to perform an SHM (Simple Harmonic Motion) before finally stopping at its original position. The spring constant is used in Hooke’s law through which we determine the SHM property. This SHM gives the concept of the constant factor of the spring. In simple words we can say that constant factor of the spring is a concept interlinked to the SHM of spring.

Spring Constant Examples

Any concept can be best understood by getting familiar with some of its problems. The solved examples given below will help you understand this concept in a crystal clear manner. By going through the given questions and their solutions, students will have a good grasp of this particular topic. Some of the solved examples on Spring constant is given hereunder.

Example 1: A given spring is elongated by 0.2 m when a force of 30 N is applied to it. Find the constant factor of that spring.

Solution: Given, the displacement in the spring, i.e., x = 0.2 m

Force (F) = 30 N 

Using the spring’s constant (k) formula, 

k = -F/x

k = -30/0.2

k = -150 N/m

The -ve sign denotes the restoring force that acts in the opposite direction of the displacement of the spring. 

Example 2: A given spring can be compressed by 0.5 m with a 100 N/m constant factor of the spring. Determine the necessary force required to perform this task.

Solution: As given in the problem, k = 100 N/m and x = 0.5 m

So using the formula for the constant factor of the spring (k) = -F/x

Putting the values of k and x in the formula, we get:

100 = -F/0.5

Ignoring the negative sign, we get

F = 50 N, this is the required force

Example 3: By applying the force of 100 N, a certain spring compresses by x meter. The value of the constant of that spring is 0.5 N/m. Find out the length by which the spring will be compressed.

Solution: We are given the value of Force and Constant factor of the spring. Here we have to determine the displacement value x.

Given, F = 100N and k = 0.5 N/m

Using the spring constant formula 

k = -F/x

Putting the given values in the formula mentioned above, we get:

0.5 = -100/x

=> x = -100/0.5

Or, x = -200 meter

That is, the spring will be compressed by 200 meters in the opposite direction of the restoring force.

Sharing is caring!