Torque Formula- Definition, Units, Applications, Examples

Torque Formula: The torque formula is one of the most important and widely used formulas in physics. This formula is primarily used to derive the value of Torque. Torque is an important concept related to the rotational motion. Torque is basically a measure of force that causes a rotational motion in an object. Its effect causes the object to rotate on its axis. Torque is the fundamental force behind all rotational events, including turning a wrench, rotating a wheel, and the Earth spinning on its axis. Let us explore the torque concepts and its formula in detail by going through solved examples.

Torque Formula

Many students find it hard to understand and apply the formula of torque correctly. It is one of the most feared topics in physics. The primary reason behind the complexity of the formula for torque is its non-linear, i.e. rotational nature. Torque is the twisting or turning effect of force and the formula of the torque is used to find the value of that twisting effect. The point on which the object rotates is known as its axis. 

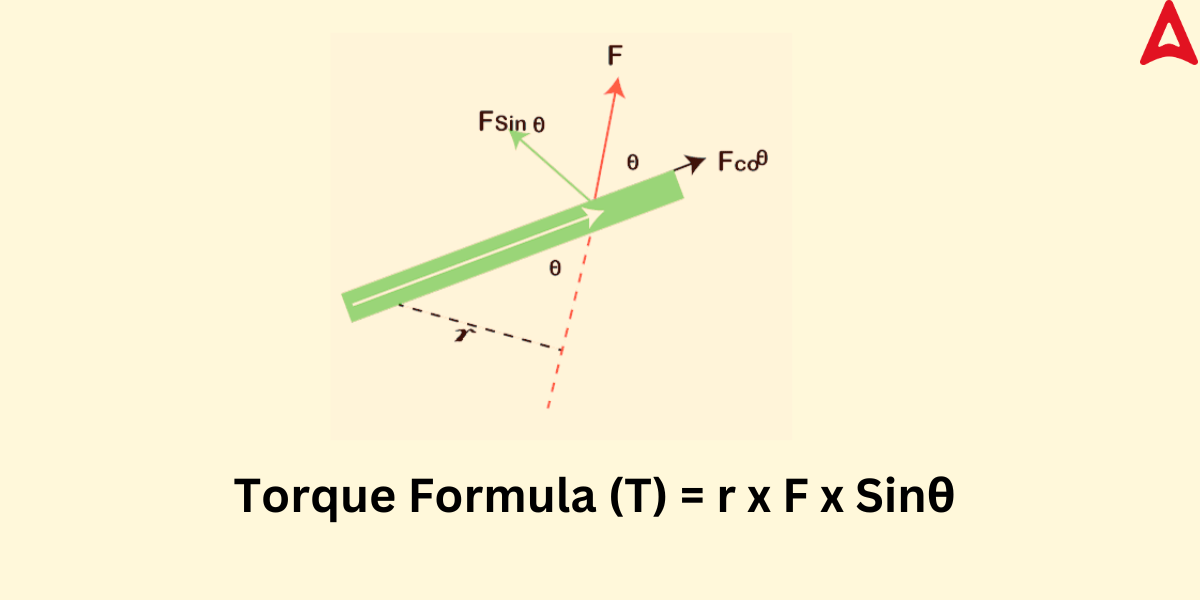
The formal formula of the torque is given by 

T = r x F x Sinθ

Where, T = Torque

F = Magnitude of force applied

r = distance of the axis of rotation from the point where F is applied

θ = angle between r and F

Must check: What is the Formula of Force?

What is Torque

Torque is simply the tendency of a force to turn or twist in physics. In linear kinematics, force is what causes an object to accelerate. Similarly, torque is responsible for angular acceleration. As a result, torque is defined as the rotating equivalent of linear force. Torque, in other terms, is the force that may cause an item to rotate around an axis.

Torque Formula Units

As we can observe from the formula for the torque mentioned above, the unit of Force is Newton (N) and the unit of distance must be in meters (m). As Sinθ is a trigonometric ratio, it has no units. So using the basic concepts of units, we get the unit of torque as the Newton meter (Nm). So the SI unit of torque is Nm.

Torque Formula in Vector Form 

Torque is a vector quantity, i.e., it has both magnitude and direction. Magnitude or direction alone cannot determine the value of torque. As torque is a vector quantity, the formula for torque can be demonstrated in the vector form. The radius vector (from the axis of rotation to the site of force application) and, consequently, the force vector are combined to generate torque in vector form. The formula of torque in vector form is given below: 

Torque = r x F, where r is the perpendicular distance vector while F is the force vector

When we apply the operation of cross product on force and radius, the formula for torque becomes:

Torque =| r | x | F | Sinθ

Where θ is the angle between the radius and force. In other words, the perpendicular component of the force, i.e. FSinθ contributes to the torque.

Relationship between Torque and Moment of Inertia

Just as Torque is the analogous physical quantity of Force in the rotational motion, so is the moment of Inertia the analogous physical quantity of mass in the rotational system. Similar to how mass determines the force required for acceleration, a rigid body’s moment of inertia, also known as the mass moment of inertia, angular mass, or rotational inertia, is a property that determines the torque required for a desired angular acceleration about a rotational axis. The formula of torque can be used to derive the relation between the moment of Inertia and Torque. The formula of torque in terms of the moment of inertia is given below:

T = I x α

Here, T = Torque,

          I = Moment of Inertia

         α = Angular acceleration

Torque Formula Applications

The formula of the torque finds its application not only in solving academic problems but also in real-life scenarios. This formula can be applied anywhere where the torque comes into play. Its effect is also associated with angular momentum and rotation. Some of the areas where the formula of torque finds its relevance are listed below.

• Vehicle Dynamics
• Machinery
• Rotational Equilibrium 
• Gear Systems
• Skating 

These are just a few real-world applications of this formula. The formula has various applications in engineering and technology.

Torque Formula Solved Examples

Learning the formula is one part, but applying it correctly is the most important aspect of learning it. Most students struggle with the correct application of the formula for torque. The solved examples given below will give you better clarity about how to apply the formula for torque.

Example 1: A door that is 1.0 m from the hinges is being pushed open by a person exerting a force of 15 N perpendicular to the door handle. Determine the torque applied to that door.

As given, F = 15 N

                R = 1.0 m

     By the torque formula T = r x F

T = 1 x 15

T = 15 Nm

So, the torque applied to the door is 15 Nm.

Example 2: A nut is loosened using a wrench. The person applies a force of 10 N at the end of the 0.6 m-long wrench handle. If the force vector and the vector of the lever arm are at a 30° angle, determine the torque delivered to the nut.

Given, 

Force (F) = 10 N

r = 0.6 m

θ = 30°

As Torque (T) = r x F x Sinθ 

T = 0.6 x 10 x Sin 30°

T = 6 x 0.5

T = 3 Nm

Example 3: A 20 m long wind turbine blade experiences a 200 N perpendicular wind force at a point 10 m from the axis of rotation. Determine the amount of wind turbine blade torque.

Given the value of Force (F) = 200 N

r = 10 m

θ = 90°

By using the formula of Torque, T = r x F x Sinθ we get,

T = 10 x 200 x Sin 90°

T = 2000 Nm

Sharing is caring!