What is the difference between a whole number and an integer?

Whole numbers include only positive numbers and zero, whereas integers include both positive and negative numbers, along with zero. In other words, integers are a superset of whole numbers.

Are fractions or decimals considered integers?

No, fractions and decimals are not considered integers. Integers are whole numbers without any fractional or decimal parts. Fractions and decimals represent numbers that are not integers.

Can negative numbers be integers?

Yes, negative numbers can be integers. Integers include both positive and negative numbers, along with zero. Negative integers are numbers less than zero.

Properties Of Integers Download Mathematics Study Notes PDF

Table of Contents

An integer is a mathematical term used to describe a set of numbers that includes positive numbers, negative numbers, and zero, without any fractional or decimal parts. Integers are a fundamental concept in mathematics and are used to represent whole numbers in various contexts.

The set of integers includes numbers such as -3, -2, -1, 0, 1, 2, 3, and so on.
The positive integers are numbers greater than zero, while the negative integers are numbers less than zero.
Zero itself is considered an integer and serves as a neutral element in many mathematical operations.

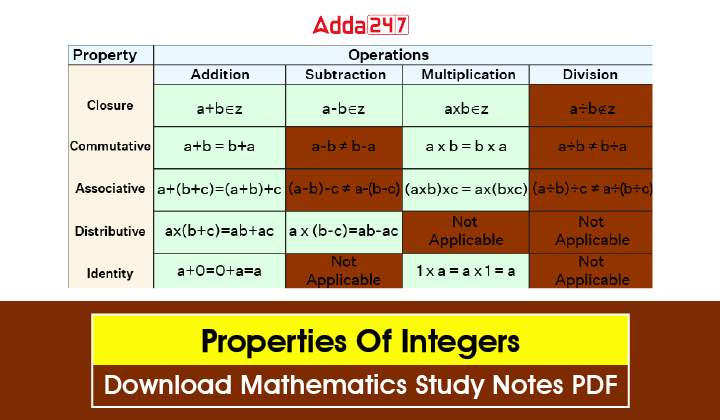
Properties of Integers

Integers possess several important properties, such as closure under addition, subtraction, and multiplication. They also exhibit properties like commutativity and associativity, which allow for consistent mathematical operations. Understanding integers is essential in many areas of mathematics and provides a foundation for more advanced concepts and calculations.

Properties of addition

Closure Property: Let a and b be any two integers, then a + b will always be an integer. This is called the closure property of addition of integers.

Examples: (a) 7 + 3 = 10

(b) (– 3) + 6 = 3

Commutative Property: If a and b are two integers, then a + b = b + a, i.e., on changing the order of integers, we get the same result. This is called the commutative property of addition of integers.

Examples: (a) 2 + 7 = 7+2 = 9

(b) (– 3) + (12) = (12) + (– 3) = 9

Associative Property: If a, b, and c are three integers, then a + (b + c) = (a + b) + c, i.e., in the addition of integers, we get the same result, even the grouping is changed. This is called the associative property of addition of integers.

Examples : [(– 3) + (– 4)] + (8) = (– 3) + [(– 4) + 8]

(– 7) + 8 = (– 3) + 4 1 =1

Additive identity : If zero is added to any integer, the value of integer does not change. If ‘a’ is an integer, then a + 0 = a = 0 + a. Hence, zero is called the additive identity of integers. Examples :

(a) 12 + 0 = 12 = 0 + 12

(b) (– 3) + 0 = (– 3) = 0 +(– 3)

Additive Inverse : When an integer is added to its opposite, we get the result as zero (additive identity). If a is an integer, then (– a) is its opposite (or vice– versa) such that a + (– a) = 0 = (– a) + a

Thus, an integer and its opposite are called the additive inverse of each other.

Examples: 2 +(– 2) = 0 = (– 2) + 2

Property of 1: Addition of 1 to any integer gives its successor.

Examples: 7+ 1 = 8 Hence, 8 is the successor of 7.

– 5 + 1 = (– 4) Hence, (– 4) is the successor of (– 5).

Properties of subtraction

Closure Property: If a and b are two integers, then a – b will always be an integer. This is called the closure property of subtraction of integers.

Examples: (a) 3 – 7 = – 4

(b) (– 5) – (– 6) = 1

Commutative Property: If a and b are two integers, then a – b b – a, i.e., commutative property does not hold good for the subtraction of integers.

Examples: 7– (– 8) = 15 but (– 8) – 7 = – 15

3 – 4 = – 1 but 4 – 3 = 1

Hence, subtraction of integers is not commutative.

Associative Property: If a, b and c are three integers, then (a – b) – C a – (b – c), i.e., associative property does not hold good for the subtraction of integers.

Example: (8– 4) – 2 = 8 – (4 – 2) , 4 – 2 = 8– 2, 2 = 6

Hence, subtraction of integers is not associative.

Property of Zero: When zero is subtracted from an integer, we get the same integer, i.e., a– 0 = a, where ‘a’ is an integer.

Examples: (a) 6– 0 = 6

(b) (– 6) – 0 = (– 6)

Property of 1: Subtraction of 1 from any integer gives its predecessor.

Examples

(a) 7– 1 = 6 (6 is predecessor of 7.)

(b) (– 3) – 1 = (– 4) [(– 4) is predecessor of (– 3).]

Properties of multiplication

Closure Property: If a and b are two integers then a × b will also be an integer. This is called the closure property of multiplication of integers.

Examples: (a) 3 × (– 4) = (– 12)

(b) (– 7) (– 2) = 14

Commutative Property: If a and b are two integers, then a × b=b × a, i.e., on changing the order of integers, we get the same result. This is called the commutative property of multiplication of integers.

Examples: (a) 7 × 2 = 2 × 7 = 14

(b) (– 3) × (– 7) = (– 7) × (– 3) = 21

Thus, commutative property holds good for the multiplication of integers.

Associative Property: If a, b and c are three integers, then a × (b × c) = (a × b) × c. This is called the associative property of multiplication of integers.

Examples: (3 × 4) × 5 = 3 × (4 × 5)

12 × 5 = 3 × 20, 60 = 60

Thus, associative property holds good for the multiplication of integers.

Multiplicative Identity: The product of any integer and 1 gives the same integer. If ‘a’ is an integer, then a × 1 = a = 1 × a.

Hence, 1 is called the multiplicative identity.

Examples: (a) 7 × 1 = 1 × 7 = 7

(a) (– 3) × 1 = 1 × (– 3) = (– 3)

Multiplicative Inverse: The product of any integer and its reciprocal gives the result as 1 (multiplicative identity). If ‘a’ is an integer, then a × = 1 = × a. Thus, an integer and its reciprocal are called the multiplicative inverse of each other.

Examples: (a) 3 × (b) (-5) × = 1 =

Property of Zero : The product of any integer and zero gives the result as zero. If ‘a’ is an integer, then a × 0 = 0 × a = 0.

Examples : 6 × 0 = 0 × 6 = 0

Distributive Property: Multiplication distributes over addition. If a, b, and c are three integers, then a × (b + c) = ab + ac. This is called the distributive property of multiplication of integers.

Examples : (– 7) × [3 + (– 4)] = (– 7) (3) + (– 7) × (– 4)

(– 7) × (– 1) = (– 21) + 28, 7 = 7